19.1 – Volatility Types
The last few chapters have laid a foundation of sorts to help us understand Volatility better. We now know what it means, how to calculate the same, and use the volatility information for building trading strategies. It is now time to steer back to the main topic – Option Greek and in particular the 4th Option Greek “Vega”. Before we start digging deeper into Vega, we have to discuss one important topic – Quentin Tarantino ☺.
I’m huge fan of Quentin Tarantino and his movies. For people not familiar with Quentin Tarantino let me tell you, he is one of the most talented directors in Hollywood. He is the man behind super cult flicks such as Pulp Fiction, Kill Bill, Reservoir Dogs, Django Unchained etc. If you’ve not watched his movies, I’d suggest you do, you may just love these movies as much as I do.
It is a known fact that when Quentin Tarantino directs a movie, he keeps all the production details under wraps until the movies trailer hits the market. Only after the trailer is out people get to know the name of movie, star cast details, brief story line, movie location etc. However, this is not the case with the movie he is directing these days, titled “The Hateful Eight”, due to be released in December 2015. Somehow everything about ‘The Hateful Eight’ – the star cast, storyline, location etc is leaked, hence people already know what to expect from Tarantino. Now given that most of the information about the movie is already known, there are wild speculations about the box office success of his upcoming movie.
We could do some analysis on this –
- Past movies – We know almost all of Tarantino’s previous movies were successful. Based on his past directorial performance we can be reasonably certain that ‘The Hateful Eight’ is likely to be a box office hit
- Movie Analyst’s forecast – There are these professional Hollywood movie analysts, who understand the business of cinema very well. Some of these analysts are forecasting that ‘The Hateful Eight’ may not do well (unlike his previous flicks) as most of the details pertaining to the movie is already, failing to enthuse the audience
- Social Media – If you look at the discussions on ‘The Hateful Eight’ on social media sites such as Twitter and Facebook, you’d realize that a lot of people are indeed excited about the movie, despite knowing what to expect from the movie. Going by the reactions on Social Media, ‘The Hateful Eight’ is likely to be a hit.
- The actual outcome – Irrespective of what really is being expected, once the movie is released we would know if the movie is a hit or a flop. Of course this is the final verdict for which we have to wait till the movie is released.
Tracking the eventual fate of the movie is not really our concern, although I’m certainly going to watch the movie ☺.
Given this, you may be wondering why we are even discussing Quentin Tarantino in a chapter concerning Options and Volatility! Well this is just my attempt (hopefully not lame) to explain the different types of volatility that exist – Historical Volatility, Forecasted Volatility, and Implied Volatility. So let’s get going.
Historical Volatility is similar to us judging the box office success of ‘The Hateful Eight’ based on Tarantino’s past directorial ventures. In the stock market world, we take the past closing prices of the stock/index and calculate the historical volatility. Do recall, we discussed the technique of calculating the historical volatility in Chapter 16. Historical volatility is very easy to calculate and helps us with most of the day to day requirements – for instance historical volatility can ‘somewhat’ be used in the options calculator to get a ‘quick and dirty’ option price (more on this in the subsequent chapters).
Forecasted Volatility is similar to the movie analyst attempting to forecast the fate of ‘The Hateful Eight’. In the stock market world, analysts forecast the volatility. Forecasting the volatility refers to the act of predicting the volatility over the desired time frame.
However, why would you need to predict the volatility? Well, there are many option strategies, the profitability of which solely depends on your expectation of volatility. If you have a view of volatility – for example you expect volatility to increase by 12.34% over the next 7 trading sessions, then you can set up option strategies which can profit this view, provided the view is right.
Also, at this stage you should realize – to make money in the stock markets it is NOT necessary to have a view on the direction on the markets. The view can be on volatility as well. Most of the professional options traders trade based on volatility and not really the market direction. I have to mention this – many traders find forecasting volatility is far more efficient than forecasting market direction.
Now clearly having a mathematical/statistical model to predict volatility is much better than arbitrarily declaring “I think the volatility is going to shoot up”. There are a few good statistical models such as ‘Generalized AutoRegressive Conditional Heteroskedasticity (GARCH) Process’. I know it sounds spooky, but that’s what it’s called. There are several GARCH processes to forecast volatility, if you are venturing into this arena, I can straightaway tell you that GARCH (1,1) or GARCH (1,2) are better suited processes for forecasting volatility.
Implied Volatility (IV) is like the people’s perception on social media. It does not matter what the historical data suggests or what the movie analyst is forecasting about ‘The Hateful Eight’. People seem to be excited about the movie, and that is an indicator of how the movie is likely to fare. Likewise the implied volatility represents the market participant’s expectation on volatility. So on one hand we have the historical and forecasted volatility, both of which are sort of ‘manufactured’ while on the other hand we have implied volatility which is in a sense ‘consensual’. Implied volatility can be thought of as consensus volatility arrived amongst all the market participants with respect to the expected amount of underlying price fluctuation over the remaining life of an option. Implied volatility is reflected in the price of the premium.
For this reason amongst the three different types of volatility, the IV is usually more valued.
You may have heard or noticed India VIX on NSE website, India VIX is the official ‘Implied Volatility’ index that one can track. India VIX is computed based on a mathematical formula, here is a whitepaper which explains how India VIX is calculated –
If you find the computation a bit overwhelming, then here is a quick wrap on what you need to know about India VIX (I have reproduced some of these points from the NSE’s whitepaper) –
- NSE computes India VIX based on the order book of Nifty Options
- The best bid-ask rates for near month and next-month Nifty options contracts are used for computation of India VIX
- India VIX indicates the investor’s perception of the market’s volatility in the near term (next 30 calendar days)
- Higher the India VIX values, higher the expected volatility and vice-versa
- When the markets are highly volatile, market tends to move steeply and during such time the volatility index tends to rise
- Volatility index declines when the markets become less volatile. Volatility indices such as India VIX are sometimes also referred to as the ‘Fear Index’, because as the volatility index rises, one should become careful, as the markets can move steeply into any direction. Investors use volatility indices to gauge the market volatility and make their investment decisions
- Volatility Index is different from a market index like NIFTY. NIFTY measures the direction of the market and is computed using the price movement of the underlying stocks whereas India VIX measures the expected volatility and is computed using the order book of the underlying NIFTY options. While Nifty is a number, India VIX is denoted as an annualized percentage
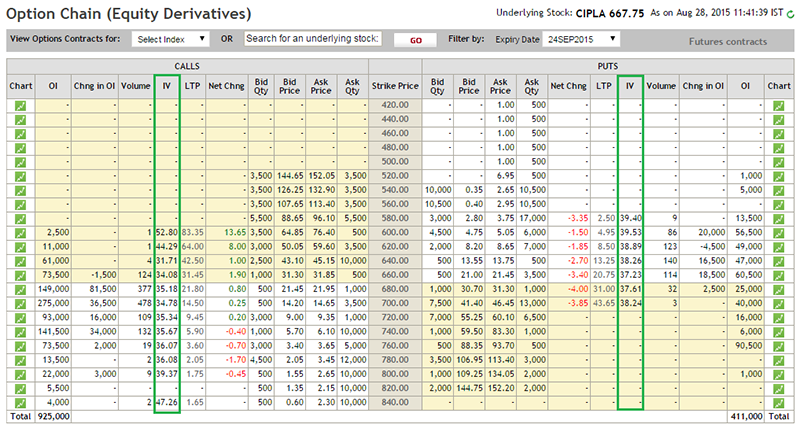
Further, NSE publishes the implied volatility for various strike prices for all the options that get traded. You can track these implied volatilities by checking the option chain. For example here is the option chain of Cipla, with all the IV’s marked out.
The Implied Volatilities can be calculated using a standard options calculator. We will discuss more about calculating IV, and using IV for setting up trades in the subsequent chapters. For now we will now move over to understand Vega.
Realized Volatility is pretty much similar to the eventual outcome of the movie, which we would get to know only after the movie is released. Likewise the realized volatility is looking back in time and figuring out the actual volatility that occurred during the expiry series. Realized volatility matters especially if you want to compare today’s implied volatility with respect to the historical implied volatility. We will explore this angle in detail when we take up “Option Trading Strategies”.

19.2 – Vega
Have you noticed this – whenever there are heavy winds and thunderstorms, the electrical voltage in your house starts fluctuating violently, and with the increase in voltage fluctuations, there is a chance of a voltage surge and therefore the electronic equipments at house may get damaged.
Similarly, when volatility increases, the stock/index price starts swinging heavily. To put this in perspective, imagine a stock is trading at Rs.100, with increase in volatility, the stock can start moving anywhere between 90 and 110. So when the stock hits 90, all PUT option writers start sweating as the Put options now stand a good chance of expiring in the money. Similarly, when the stock hits 110, all CALL option writers would start panicking as all the Call options now stand a good chance of expiring in the money.
Therefore irrespective of Calls or Puts when volatility increases, the option premiums have a higher chance to expire in the money. Now, think about this – imagine you want to write 500 CE options when the spot is trading at 475 and 10 days to expire. Clearly there is no intrinsic value but there is some time value. Hence assume the option is trading at Rs.20. Would you mind writing the option? You may write the options and pocket the premium of Rs.20/- I suppose. However, what if the volatility over the 10 day period is likely to increase – maybe election results or corporate results are scheduled at the same time. Will you still go ahead and write the option for Rs.20? Maybe not, as you know with the increase in volatility, the option can easily expire ‘in the money’ hence you may lose all the premium money you have collected. If all option writers start fearing the volatility, then what would compel them to write options? Clearly, a higher premium amount would. Therefore instead of Rs.20, if the premium was 30 or 40, you may just think about writing the option I suppose.
In fact this is exactly what goes on when volatility increases (or is expected to increase) – option writers start fearing that they could be caught writing options that can potentially transition to ‘in the money’. But nonetheless, fear too can be overcome for a price, hence option writers expect higher premiums for writing options, and therefore the premiums of call and put options go up when volatility is expected to increase.
The graphs below emphasizes the same point –
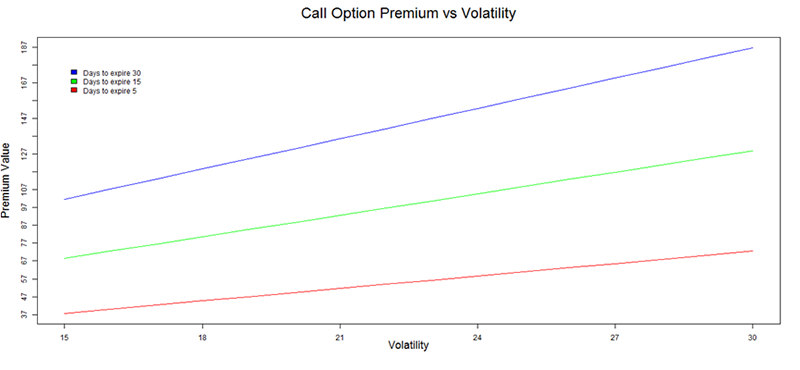
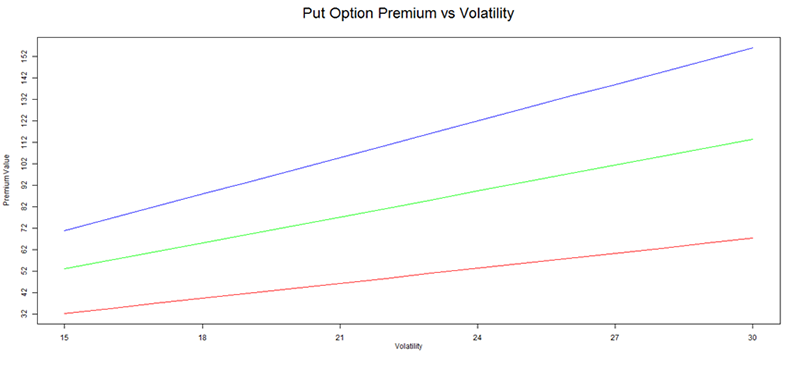
X axis represents Volatility (in %) and Y axis represents the premium value in Rupees. Clearly, as we can see, when the volatility increases, the premiums also increase. This holds true for both call and put options. The graphs here go a bit further, it shows you the behavior of option premium with respect to change in volatility and the number of days to expiry.
Have a look at the first chart (CE), the blue line represents the change in premium with respect to change in volatility when there is 30 days left for expiry, likewise the green and red line represents the change in premium with respect to change in volatility when there is 15 days left and 5 days left for expiry respectively.
Keeping this in perspective, here are a few observations (observations are common for both Call and Put options) –
- Referring to the Blue line – when there are 30 days left for expiry (start of the series) and the volatility increases from 15% to 30%, the premium increases from 97 to 190, representing about 95.5% change in premium
- Referring to the Green line – when there are 15 days left for expiry (mid series) and the volatility increases from 15% to 30%, the premium increases from 67 to 100, representing about 50% change in premium
- Referring to the Red line – when there are 5 days left for expiry (towards the end of series) and the volatility increases from 15% to 30%, the premium increases from 38 to 56, representing about 47% change in premium
Keeping the above observations in perspective, we can make few deductions –
- The graphs above considers a 100% increase of volatility from 15% to 30% and its effect on the premiums. The idea is to capture and understand the behavior of increase in volatility with respect to premium and time. Please be aware that observations hold true even if the volatility moves by smaller amounts like maybe 20% or 30%, its just that the respective move in the premium will be proportional
- The effect of Increase in volatility is maximum when there are more days to expiry – this means if you are at the start of series, and the volatility is high then you know premiums are plum. Maybe a good idea to write these options and collect the premiums – invariably when volatility cools off, the premiums also cool off and you could pocket the differential in premium
- When there are few days to expiry and the volatility shoots up the premiums also goes up, but not as much as it would when there are more days left for expiry. So if you are a wondering why your long options are not working favorably in a highly volatile environment, make sure you look at the time to expiry
So at this point one thing is clear – with increase in volatility, the premiums increase, but the question is ‘by how much?’. This is exactly what the Vega tells us.
The Vega of an option measures the rate of change of option’s value (premium) with every percentage change in volatility. Since options gain value with increase in volatility, the vega is a positive number, for both calls and puts. For example – if the option has a vega of 0.15, then for each % change in volatility, the option will gain or lose 0.15 in its theoretical value.
19.3 – Taking things forward
It is now perhaps time to revisit the path this module on Option Trading has taken and will take going forward (over the next few chapters).
We started with the basic understanding of the options structure and then proceeded to understand the Call and Put options from both the buyer and sellers perspective. We then moved forward to understand the moneyness of options and few basic technicalities with respect to options.
We further understood option Greeks such as the Delta, Gamma, Theta, and Vega along with a mini series of Normal Distribution and Volatility.
At this stage, our understanding on Greeks is one dimensional. For example we know that as and when the market moves the option premiums move owing to delta. But in reality, there are several factors that works simultaneously – on one hand we can have the markets moving heavily, at the same time volatility could be going crazy, liquidity of the options getting sucked in and out, and all of this while the clock keeps ticking. In fact this is exactly what happens on an everyday basis in markets. This can be a bit overwhelming for newbie traders. It can be so overwhelming that they quickly rebrand the markets as ‘Casino’. So the next time you hear someone say such a thing about the markets, make sure you point them to Varsity ☺.
Anyway, the point that I wanted to make is that all these Greeks manifest itself on the premiums and therefore the premiums vary on a second by second basis. So it becomes extremely important for the trader to fully understand these ‘inter Greek’ interactions of sorts. This is exactly what we will do in the next chapter. We will also have a basic understanding of the Black & Scholes options pricing formula and how to use the same.
19.4 – Flavors of Inter Greek Interactions
(The following article was featured in Business Line dated 31st August 2015)
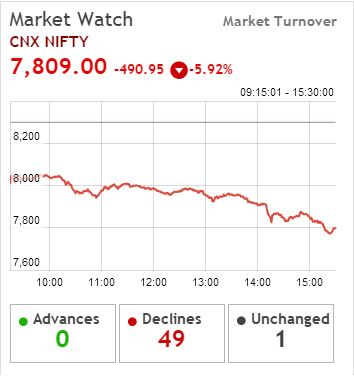
Here is something that happened very recently. By now everyone remotely connected with the stock market would know that on 24th August 2015, the Indian markets declined close to 5.92% making it one of the worse single day declines in the history of Indian stock markets. None of the front line stocks survived the onslaught and they all declined by 8-10%. Panic days such as these are a common occurrence in the equity markets.
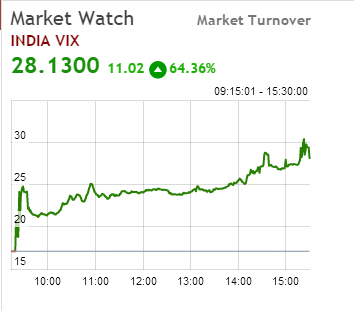
However something unusual happened in the options markets on 24th August 2015, here are some data points from that day –
Nifty declined by 4.92% or about 490 points –
India VIX shot up by 64% –
But Call option Premiums shot up!
Traders familiar with options would know that the call option premiums decline when market declines. In fact most of the call option premiums (strikes below 8600) did decline in value but option strikes above 8650 behaved differently – their premium as opposed to the general expectation did not decline, rather increased by 50-80%. This move has perplexed many traders, with many of the traders attributing this move to random theories such as rate rigging, market manipulation, technological inefficiency, liquidity issues etc. But I suspect any of this is true; in fact this can be explained based on the option theory logic.
We know that option premiums are influenced by sensitivity factors aka the Option Greeks. Delta as we know captures the sensitivity of options premium with respect to the movement of the underlying. Here is a quick recap – if the Delta of a particular call option is 0.75, then for every 1 point increase/decrease in the underlying the premium is expected to increase/decrease by 0.75 points. On 24th August, Nifty declined by 490 points, so all call options which had ‘noticeable Delta’ (like 0.2, 0.3, 0.6 etc) declined. Typically ‘in the money’ options (as on 24th Aug, all strike below 8600) tend to have noticeable Delta, therefore all their premiums declined with the decline in the underlying.
‘Out of the money’ options usually have a very low delta like 0.1 or lower. This means, irrespective of the move in the underlying the moment in the option premium will be very restrictive. As on August 24th, all options above 8600 were ‘out of the money’ options with low delta values. Hence irrespective of the massive fall in the market, these call options did not lose much premium value.
The above explains why certain call options did not lose value, but why did the premiums go up? The answer to this is lies in Vega – the option Greek which captures the sensitivity of market volatility on options premiums.
With increase in volatility, the Vega of an option increases (irrespective of calls and puts), and with increase in Vega, the option premium tends to increase. On 24th August the volatility of Indian markets shot up by 64%. This increase in volatility was totally unexpected by the market participants. With the increase in volatility, the Vega of all options increases, thereby their respective premiums also increased. The effect of Vega is particularly high for ‘Out of the money’ options. So on one hand the low delta value of ‘out of the money’ call options prevented the option premiums from declining while on the other hand, high Vega value increased the option premium for these out of the money options.
Hence on 24th August 2015 we got to witness the unusual – call option premium increasing 50 – 80% on a day when markets crashed 5.92%.
Key takeaways from this chapter
- Historical Volatility is measured by the closing prices of the stock/index
- Forecasted Volatility is forecasted by volatility forecasting models
- Implied Volatility represents the market participants expectation of volatility
- India VIX represents the implied volatility over the next 30 days period
- Vega measures the rate of change of premium with respect to change in volatility
- All options increase in premium when volatility increases
- The effect of volatility is highest when there are more days left for expiry


Hello Sir,
I was just studying the nifty50 chart on 25th July 2025 which shows a sharp Gap Down open and after that chart went down, which should reflect in option chain and It was. All ITM, ATM, and some OTM CE option was down but Deep CE OTM has given a profit upto 64-74 % . Is it the same reason that as the incident of 24th aug 2015 you mentioned above ?
And one more thing all the Deep OTM for put options was showing losses of 12-16 %.
Please Reply
Ayush, I\’ve not seen the option chain, but yes, premiums are not just dependent on the directional moves but also dependent on the volatility. So do make sure to check volatility and how that is impacting the option premium.
And why deep OTM PE showing loss I could not figure out?
Because its deep OTM 🙂
See there are various factors (greeks) at play, each exhibiting its own force on the premium. Some forces have bigger impact considered to others.
Got your Point, Thanks
Happy learning!
on 7th April 2025, entire trading fraternity knows what happened. Nifty fell more than 1000 points and Vix changed by 67%. According to your findings the call options of April Expiry should had increased. But it did not happen.
Request you to discuss the happenings on 7th April 2025.
The effect of increase in Vix is offset by the the change in delta, hence CE wont increase 🙂
Hi Karthik,
Thanks for amazing explanation provided above. Like you mentioned that due to increasing Vega and non existent delta for CE OTM options, sometimes when the underlying falls, the CE premium increase. However, I have also noticed that sometimes India VIX and the underlying both fall, however the CE premium still rises. Can you please explain this scenario.
Mayank, if both underlying price and vix falls, I\’m not sure if the CE premium increases. Unless there was a change in interest rate at the same time.
Thanks for your response Karthik, I am not able to share screenshot here, but the details of the scenario, I am talking about are mentioned below. If you can check this respond, I would be very grateful
Underlying: Bank Nifty
Option: Bank Nifty 18th SEP 52800 CE
Date: 12 September 2024
India IVX closed lower at 15:27 PM compared to 15:19 PM
Bank Nifty closed lower at 15:27 PM compared to 15:19 PM
Bank Nifty 18th SEP 52800 CE closed higher at 15:27 PM compared to 15:19 PM
Mayank, not fully getting the context. But I guess you have to see the difference between the settlement price (since its expiry) as opposed to closing price.
Hi Karthik,
For
Nifty Strike=23500
Expiry=2025-03-27 15:30:00
NSE website gives
Call Option IV=13.21
Put Option IV=17.02
and Sensibull gives
IV=13.4
Can you please confirm the reason/s behind these differences? Thank you.
Not sure, I think you will have to speak to Sensibull for the IV calculation methodology.
Would love your take on predicting volatility, especially using GARCH or any other model
Thats highly quantitative Bala. I remember we had posted an article around this, forgot which platform. Will share as soon as I find 🙂
Does premiums change only due to change in implied volatility or also because of changes in realized volatility?
Implied volatility. Realized volatility is backward looking, while implied is forward looking.
Hi karthik,Really appreciate your effort of making things understandable & sharing a valuable knowledge.I have understood the concept.But i have confusion,How can we know that at the start of the month volatility is high or low.India VIX is trading in different ranges across different months.Do we have any indication like RSI,which gives clear picture like over bought Or oversold.can you please give some detailed undersatnding on this.Thanks in advance
One easy way is to look at historical volatility and getting a sense of where Vix is today. Gives a quick perspective.
Double diagonal is positive vega strategy while iron condor is negative vega. Which strategy is good in which type of market and when to deploy them ?
Thanks.
Every startegy has its day in the market, based on the market situation. One strategy does not fit all days 🙂
Sir,
Based on what you taught in volatility section to find the range of transition for a stock during a particular period, I have prepared a small Mathematical analysis. I would like you to comment if the below could help while placing Trades (spot/Future).
Scenario: Suppose that a particular stock is falling from its high from 01.01.2020 (stock price-70) to 07.01.2020 (stock price-67).
(i) On 07.01.2020, it has given a hammer at the low with good volume and support.
(ii) The stock is backd by good fundamentals.
(iii) Annual realized volatility for 7 days calculated till 31.12.2019 is 5%. 7 day average return is 3%. Hence, lower range return 3%-5%=-2%. Thus, in general stock price on 07.01.2020 should have minimum value for 70-70*2%=68.6. However, the stock price on 07.01.2020 is 67 (black swan event).
(iv) Besides, annual realized volatility on 07.01.2020 is greater than calculated 1D annual realized volatility since the past 30 days till 06.01.2020.
Can I imply here that this type of scenario can indicate Mathematically (along with Technical & good fundamental) that the price can move upward?
Sir,
I lack the system which you have shown in Futures for Pair trading etc. Hence, I mean to ask that will it be advisable if I place trade in futures based on Technical (Directional) & taking Fundamental along with it?
Yes, but that wont be basis a pair trade, it will be a TA driven trade.
Sir,
You have discussed pair trading and few techniques in futures.
My query- Is it advisable to place trades in futures merely based on Technical analysis? Your viewpoint pls?
Ppl do that, but my advice is to keep fundamentals also in perspective.
Sir,
Is there any mathematical analysis available which will indicate if the price of a certain stock for the upcoming 1 min/1 hr/1 day/1 week is likely to make positive or negative returns?
Ah, dont think so 🙂
Sir,
Just to receive your opinion on the below:
You have shown to find range of swing of a stock/index thorugh collecting each day\’s close data and then chalk out mean, s.d and then ultimately find s.d for a day. Accordingly we then find range for a particular time frame.
(i) Will it be more accurate if we would rather collect historical data hourly basis (rather than day) data and then find range for a particular time frame?
(ii) Also, if we want range for intraday/hourly/minute time frame, will it be more accurate if we collect and consider only recent data say, last 30 days (and not the entire last year)? I am a little perplexed here.
1) Too much data can lead to noise and not necessarily good information. Thats my belief, I\’ve not backtested it. You can maybe backtest and see if you get good results with hourly data. Do share your results 🙂
2) Yes, that seems intuitive.
Sir,
In option chain, I could see \”OPEN PRICE,HIGH PRICE,LOW PRICE,CLOSE PRICE,LAST PRICE,SETTLE PRICE\” indicates?
Its the OHLC of the option premium, just like the way you\’d find for stocks.
Sir,
Suppose, on one day, India VIX value of 16. I hope it means for a year ahead. Suppose again that the time to expiry is 12 days. Then if I want to measure the volatility for 12 days , can we move ahead like – 16/sqrt 252*12
ViX is annualized, yes, you can convert it to whatever time you need by dividing it by Sq root of time.
Sir,
Like volatility helps in prediction of range for the underlying, does implied volatility figure also helps to realize the range of the underlying movement? If no,does it helps if realizing the premium range of options? I am a bit confused.
Nope, not really. But then end of the day, it is a volatility type. So it does help to some extent.
Sir,
Does technical analysis hold good in graph of Implied volatility?
Nope, not at all. Implied volatility is derived and not an asset or an underlying.
Sir,
In one area, you wrote that realized volatility is required when we try to compare today\’s implied volatility over historical implied volatility. Could you kindly elaborate here?
Today\’s realized volatility is tomorrow\’s historical volatility. So all volatility types are related 🙂
Sir,
The implied volatility changes on every day.That means the prediction of volatility for an upcoming period also changes every time. Right?
That\’s right, with change in prices, the IV also changes.
Sir,
What is the implied volatility that is published in NSE suggest? Does that suggest volatility for an upcoming month time or is it annualized?
Its based on the ATM CE and PE premium – what the market expects for the next 30 days.
Sir,
I am finding a bit difficulty in understanding the subtle differences of various types of volatility that you discussed. In between tried to get help of some material in net. Could you kindly help me with the below if I am correct?
(i) Historical volatility, Forecasted volatility and realized volatility- All are measured with respect to underlying movement.
(ii) Implied volatility- Meausred with respect to option premium pricing.
Thats correct. I think I had given a weather example to explain this, did you get a chance to see that?
Hello,
In this statement:
\”The Vega of an option measures the rate of change of option’s value (premium) with every percentage change in volatility\”.
Does \”Volatility\” here refer to the Implied Volatility or the Realized Volatility?
It refers to the implied volatility.
Dear Sir,
If the INDIA VIX is 16, does it mean that the volatility anticipated for the next 30 calendar days is 16 percent from the current value? or is it indicating an annual change of 16 percent from the current value?
\”India VIX indicates the investor’s perception of the market’s volatility in the near term (next 30 calendar days)\”
\”While Nifty is a number, India VIX is denoted as an annualized percentage\”
– I am slightly confused with the above statements. Many thanks in advance
Its 16% annual, you will have to scale it down to the time frame you are interested in looking at.
Sir.. I am nither a programmer nor a statics student… Can i forecast volatility by using GARCH model?? If yes then how?? If no then what is required to do this?? Also suggest me some study material where I can understand GARCH model in a lucid manner..
That would be really tough. But maybe you can try on Excel.
Dear Mr. Karthik, Thank you very much for your prompt response. Basically, I was comparing your statements as stated in the module against 2 other sources. There is a difference in terms of the Vega value for OTM options. Thank you
Sure, good luck John. I\’ll dig this again as well 🙂
Dear Mr. Karthik,
Thank you very much for sharing with us so many of your in-depth and informative articles on Options. To date, you have been the only writer who has been able to provide very unique perspective on Options from a practical approach.
Regarding your following 2 statements in your Modules on Vega:
“The effect of Vega is particularly high for ‘Out of the money’ options”
“High Vega value increased the option premium for these out of the money options”
However, based on the following 2 sources (as attached below), Vega is lowest for OTM and ITM which is different from your above-mentioned statement.
I seek your kind enlightenment.
Thank you
https://www.macroption.com/option-vega/
At the money options have greatest time value and highest vega. Options further away from the money to either side (ITM, OTM) have less time value and lower vega.
https://www.merrilledge.com/investment-products/options/learn-understand-vega-options
Vega is centered around ATM options and falls as it moves OTM or ITM.
Thanks John. Let me review my content again, but these are graphs inferred from B&S model, so I\’m fairly certain about it, but no harm double checking i guess 🙂
Just let me know what are the statistical models are used for predict price of an instrument?
There are many, Suman. One of the most common method involves regression models.
but I could not find trailing sl for delivery trades in kite app
You can mentally trail the stoploss.
Hello karthik sir,
As an aspiring trader, market participant and a varsity learner , my job is to find oppurtunities in the markets, identify the probability of risk and reward (like for bullish position , we need to find the stocks that are in downtrend and a reversal candlestick pattern like bullish engulfing/morning star and hammer with good volumes and take the long trade with determined target and stoploss. But when after exiting , reaching the target price, the stock moves up a lot. If I book profit or book a loss, I am happy but when I see it is going up even after reaching the target, it\’s causing some pain in the mind disturbing the mindset while taking trades, decreasing the confidence level. How to handle this ?For example , I bought voltas for a swing trade at 797 , sold at 870 with a risk to reward of 1:10 now it is above 900rs within a month. It happened with me in several stocks like idfc first bank, irfc , syrma sgs.
I totally understand and it does happen to all of us. One easy way to deal with this is to deploy something called as a trailing stop loss, where you trail your profits and not sell everything at once. Keep pushing the stop loss and let the position move along.
Thank you for putting in so much effort in designing these learning modules. I am a newbie to the markets and have learnt immensely.
Glad to note that, Nandan. Happy learning 🙂
Thanks. That is what I was trying to infer – if Put IV is higher, there is higher Put buy demand vis-a-vis Call buy demand generally by bigger hands, isn\’t it? Conversely or another way to look at it, bigger hands are interested in selling calls aggressively vis-a-vis Puts. I know nothing is certain in the markets, but isnt this the likely scenario that causes this IV or pricing imbalance?
Yes, these are demand-supply forces at play, Jatin and they do tend to impact the IV. Btw, another factor to consider is the liquidity of the contract as well. Lower the liquidity, higher the IV.
Hi Karthik – I cannot thank you enough for the great content, presented in a super-simple format. I have a very lame and basic doubt. I have seen several times in the option chain that the IV for the call and put options vary significantly for same (ATM) strike price or equidistant OTM options. e.g. today, 19th Jul, 10.35 am, below are IVs. If, say, Put IVs are relatively much higher than Call IVs for equidistant OTM strikes, can we assume that it is \’likely\’ that fear is higher on that side (e.g. Put in this case), and hence, market unlikely to go up? If not, what could be the reason for such large differences?
Nifty Spot – 19806
19800 Call – IV – 13.96
19800 Put – IV – 16.3
20000 Call IV – 14.07
19600 Put IV – 19.04
Thanks Jatin, glad you liked the content. Each strike has a its own demand and supply situation, which tends to impact the strike specific IVs, hence the difference.
what is GARCH model??
can you please provide some insights.
Its a statistical method used to make volatility predictions.
hello karthik,
nice article . can you help me with relation of strike prices and volatility. as you mentioned at OTM options volatility was high
Nikhil, I have discussed this in detail in the later chapters. Request you to kindly check that. Thanks.
Hello karthik sir!!!
Vega is generally highest and lowest for which type of options- OTM,ATM,ITM ?
Also, with the increase in volatility, vega increases the most and least for which type of option- OTM,ITM, ATM ?
Closer to expiry, it\’s for ATM strike. Also, with an increase in volatility, its always the ATM which reacts the most. Do check the chapter, I\’ve discussed this in detail.
Karthik Sir
why implied volatility of options (of nearby expiry date ) increases near expiry date ( when compared to far away expiry ) & highest on expiry date ?
Karthik Sir ,
why implied volatility of options increases near expiry date ( when compared to options with far away expiry date ) & highest on expiry day ?
thank you
The non-mathematical explanation is that as expiry approaches, there is greater uncertainty (especially for ATM options) to transitioning to OTM or ITM. Hence the volatility tends to increase.
Sir you have said IV of option strikes can be calculated, please tell how that is done, or maybe i misses something.
As I mentioned, this can be done using the B&S calculator.
Sir u have IV of option strikes can be calculated, please tell how that is done, or maybe i a missed something here.
You\’ll need to use a B&S calculator for this Sagar.
As IV is usually more valued, isn\’t it a good idea to use Implied Volatility (IndiaVIX) to use for the calculation of the underlying\’s range for \’x\’ number of days? Instead of Historical Volatility?
You need a time series of volatility, that\’s why we look at historical vol.
And oh, did I mention this – Amazing content so far. This is the 5th module I am reading and I must say all these modules are very well written and easy to grasp. I can only imagine the sheer work that the author has put in to simplify and organise everything. Wonderful !!!
Thanks for the kind words, I hope you continue to enjoy learning on Varsity!
Everything being said, one important thing to note here is – Quentin Tarantino, getting a lifetime pass for directing Pulp fiction.
Hahah, I agree 🙂
Hi Kartik, In section 19.2 towards the end, you have written that, ‘for each % change in volatility the option will gain or lose 0.15 of its theoretical value’. It implies that the option value may decrease too. How is this possible if vega is always positive. The gain/lose mentioned above does it pertain to value of vega or premium value of the options.
So vega is positive, if the volatility increases, so does the option premium and if the volatility decreases, so would the option premium. For example, volatility moving from 15% to 20% increases the option premium and a decrease from 20% to 16%, which tends to lower the option premium.
Sir,
You said\”to conclude that the present IV for a particular stock is high or low,you need to calculate daily historical volatility and convert it to annual volatility and compare.\”
Sir instead of calculating it by ourselves through Excel,is it ok to use the ones given in NSE website,is there accuracy or any other issues with it
2.sir i donno whether this question makes sense or not,why Implied Volatility in the options chain is Annualized 1 SD if it is the volatility that the market expects over the next few trading sessions
You can actually look at the ATR indicator to get a sense of where the volatility is. That is how volatility is measured 🙂
Sir,
1.daily SD is also the realized volatility and Implied Volatility in the options chain is Annualized 1 SD
Sir,are these statements true ?
How come these are calculated in terms of SD?and also Sir,what is meant by intrinsic volatility?
2.\”volatility based on how much high/low stock went from opening price is intraday volatility. There are quant models to forecast that.It can be looked upon as a method to predict the intraday volatility.\”
Sir,is this true?if yes
How come one know a day\’s high/low until the close of the day?So how does forecasting intraday volatility works?
3.And also,on 24th August 2015 the decline in options premium wasn’t proportional to the decline in spot due to the fact that All options increase in premium when volatility increases,is it correct,sir?
1) Implied volatility is the volatility that the market expects over the next few trading sessions
2) Yes. Your models will consider the previous day\’s high and low and predict intraday vol for today
3) Yes, that\’s correct.
Sir,searched throughout the chapter as you told,but couldn\’t find answer for the question \”Why the effect of Vega is particularly high for ‘Out of the money’ options.\”is it because they have low delta.Sir,Can you please help me with this
And also,on 24th August 2015 the decline in options wasn\’t proportional to the decline in spot due to increase in volatility which eventually increases premium,Am i correct?
Yes, lower delats, and also low probability of expiring ITM. Do check my previous comment.
Sir,
Is it possible to delete or edit a comment?
Dont think you can.
Sir,
This question is in relation to the query i just raised,
\’when calculating using the option calculator vega is higher for OTM compared to deep OTM.\’
But sir,as deep OTM options have lower data,they decline less than OTM options,hence more rise.
Is it so,sir?
You can also think about this from probability perspective. Deep ITM or deep OTM have higher degree of certainty in terms of expiring ITM and OTM respectively. So change in volatility (unless its super large change) does not really impact the outsome of these options. But on the other hand, change in vega impacts the borderline options i.e. ATM and in and around ATM options.
Sir,
Why the effect of Vega is particularly high for ‘Out of the money’ options. And also did the rise in volatility limited the decline in call options strike below 8600 to any extend
Hmm, we have discussed this in the chapter itself no?
Ok sir got it. Thanks.
Good luck!
Sir, suppose I have bought 17600 CE and the volatility of PE side increases because more people are now feeling bearish.
Then should I exit my position immediately and go with people\’s perception or wait with the hope that Nifty will go up?
Please advice.
Sandeep, that\’s a tricky call to be a contrarian or go with the crowd. It depends on your reading of the market and how convinced you are on the outcome. Very hard for me to advice you on what you should do 🙂
Thank you very much sir.
Happy learning!
Sir, then what could be the reason for demand and supply increasing for this PUT side IV?
As you say that such events occur often, generally speaking why does demand and supply increase for one side IV of the same strike
as compared to the other side?
Thanks
If traders feel bearish over the next few days, then they can probably buy and sell more PUTS, thereby increasing the OI for puts, and hence the demand-supply dynamics would impact the IV.
Sir, many thanks for your explanation. I have some doubts..
1. In this case since the PUT side IV has gone up, can we say that more people are expecting price reversal?
2. Do such events occur frequently where IV\’s of the same strike are different?
1) Cant really as there are both buyers and sellers of options
2) Yes
No sir, actually I am curious as to why the CALL side IV decreased while PUT side IV of the same strike INCREASED.
What could be the possible reason? Kindly guide on that.
So one of the contributing factors for IV change is also the demand and supply dynamics. In fact, this is the reason why IVs for the same strike can be different.
Sir, many thanks for your explanation. But I have some doubts.
1. You have taught that when volatility increases, premium of BOTH CALL and PUT increase. But in this case what could be the reason
that the CALL side IV fell whereas the PUT side IV increased?
2. What is the significance of Strike specific volatility? How is it used in trading? Kindly guide sir.
1) That is true, assuming all other greeks are constant. But in reality, all greeks move in real-time. While one tends to increase the premium, others drag them down.
2) You can check the option chain from Sensibull. Its like the volatility of a particular strike; the premium will change based on how the volatility impacts that strike.
Sir, I got it why CALL premium fell despite Nifty going up. But why did the PUT increase?
As per my understanding,
– Nifty\’s volatility is common to both CALL and PUT.
– Time to expiry is same for both.
So what caused PUT to increase? Kindly guide sir.
Look at strike-specific volatility. For example, 17400 CE vol could be 22%, but 17400 PE can be 30%. PE strike volatility must have shot up, there could be no other reason.
Sir, this is the query I had posted —-> (This is from a guy\’s comment above)
1. Nifty is INCREASING.
2. Long CALL and long PUT of Slightly OTM strike and difference between two strikes is 100.
3. Although Nifty is moving in upward direction CALL premium is falling and PUT premium is increasing.
This is quite puzzling because if volatility is increasing both the CE and PE premiums should increase and if volatility is decreasing then both
CE and PE premiums should fall. Please help me understand sir why is this happening.
Got it. So the premium is not the function of directional movement. It is a function of time to expiry, volatility, the speed at which the market is moving etc. So, in this case, while the market\’s direction worked in the trade\’s favor, other factors may not play out well.
Couldn\’t get you sir. What is the effect if CE is OTM? could you please explain?
SOrry, lost the context. Can you post the complete query again, Sandeep?
Sir, time for expiry is the same for both CE and PE option. Both are slightly OTM strikes. Volatility is same for both.
But what\’s astonishing is that while underlying is increasing, the CE is decreasing and PE is increasing. What could be
the possible reason for this ?
Check the monenyess of both options. Most likely, the CE is OTM.
Dear Karthik sir,
In one of the comments above a guy has observed on someday ->
1. Nifty is INCREASING.
2. Both CALL and PUT Strikes are slightly OTM.
3. Even if Nifty is moving in upward direction CALL premium is falling and PUT premium is increasing.
What could be the reason for this? Please guide.
Thanks
That depends on many factors. Time to expiry, which strike etc.
Many thanks sir. Got it.
Happy learning!
No sir I only wanted to know that what perspective we can arrive at if we see
1. Current volatility of the stock/index is more than it\’s historical volatility? and
2. Current volatility of the stock/index is less than it\’s historical volatility? Please guide.
Thanks
Ok. If the current IV is say 23% and the historical is 24%, then the current IV is lesser than the historical, and we can expect it to go higher hence look for option buying opportunities.
Thank you so much sir. Yesterday Nifty\’s historical volatility was 22.86% and current volatility was 23%.
This means that current volatility was greater than historical volatility. Sir, what according to you it indicates when
1. Current volatility is greater than historical volatility?
2. Current volatility is less than historical volatility?
Please guide. Thanks.
Sandeep, 22.86% is as good as 23% 🙂
Sir, many thanks for your help. Today i checked the Nifty ATM\’s IV. On the CALL side, it was 22.73 while on the PUT side it was 24.88.
So which IV should we consider CALL side or PUT side?
Thanks.
Although the IV of both CEs and PEs are supposed to be similar, they don\’t due to the demand and supply mismatch. YOu can take these as a rough estimate, maybe average too works. In this case, I\’d consider the IV around 23-24%.
Thank you sir, but how to know a stock/index\’s current volatility? Kindly guide.
The easiest way is to look at the ATM\’s implied volatility.
Karthik sir,
In one of the comments a guy asked you ->
\”How to conclude that the present IV for a particular stock is high or low? Is it based on vega?\”
You said ->
For this you need to calculate daily historical volatility and convert it to annual volatility and get a “quick” perspective.
My question is with what this annual historical volatility has to be compared to get a quick perspective?
Sandeep, you compare the stock or the index\’s current volatility with its historical volatility to get a sense of how the volatility is moving.
Karthik sir, i read the comment. Quoting you….\”yes, deep OTM options do react to change in volatility – but do bear in mind this has to be a massive change in volatility, like what happened on 24th August. However, such events are not very frequent\”
So this is what I understood —> In normal cases when Volatility increases, the slight OTM reacts more than the deep OTM. Am I right sir?
Yup, that right.
Dear Karthik sir,
The chapter says \”The effect of Vega is particularly high for ‘Out of the money’ options.”
Out of slight OTM and deep OTM, which one reacts more?
Have posted the explanation in one of the earlier comments, Sandeep. Request you to check that out once.
Thanks a lot sir for helping me.
Happy learning!
I want to calculate the realized volatility for today considering today\’s OHLC values. I DON\’T want to take previous closing prices into account.
In such situation can we calculate today\’s realized volatility?
You can calculate the SD of the price. On excel = STDEV(open price, close price). But this won\’t be accurate, as SD is on returns, not price. For return, you need previous close data.
Sir, what you say is predicting the intraday volatility but I asked about calculating the volatility after market closes.
Like how much high/low stock went from opening price. Is that possible? if yes what is the method to do that.
Thanks
Ah, that is the same as historical volatility.
Actually I thought we can calculate the volatility based on how much high/low stock went from opening price. Can we do that sir?
Yes, that\’s intraday volatility. There are quant models to forecast that, but quite complex to develop one. I\’ve never done that.
Thanks sir, you have already taught that method of calculating the daily SD based on the previous closing prices.
But I wanted to know calculating the daily volatility based on the OHLC of any particular day, suppose today.
Is there any such method ?
Thanks
Both are the same no, Sandeep?
Thanks sir.
1. How do we know what was the realized volatility for a stock after market closing?
2. I have gone through the chapters of historical volatility but what is the calculation for realized volatility for a particular day?
Thanks.
1) On a closing basis, you can calculate the SD with respect to the previous few closing. This will give you the daily SD, which is also the realized volatility
2) Its the same.
Got it sir. So realized volatility is another name for Historical Volatility that we learnt in Chapter 17 and 18 right?
Yes, thats right. Today\’s realised volatility becomes the historical volatility.
Dear Karthik sir,
How can we know the Realized volatility of a stock? Thanks
Look at the historical volatility, you can calculate that on excel as well.
And the Intrinsic Volatility in the options chain is 1 SD right sir?
Yes.
Dear Karthik sir,
The Implied Volatility that we see in the options chain is Annualized 1 SD right? Thanks
Yes, its annualised.
Ok sir kindly put a video on this soon.
Dear Karthik sir,
Is India VIX helpful in trading? If it is, kindly explain how to use it effectively in trading.
Thanks
This is slightly complicated, I will try and put up a video on this.
Dear Karthik sir,
Please solve these queries of mine :
1. When the volatility increases then only the Premiums for CE options increase or both CE and PE increase ?
Similarly,
2. When the volatility decreases then only the Premiums for PE options decrease or both CE and PE decrease ?
Thanks
1) Both CE and PE will increase
2) Both
All else equal, only if Volatility increases option premiums increase, likewise with the decrease in volatility, premiums decline too.
Thanks a lot sir for your explanation. 🙂
Dear Karthik sir,
The chapter says that Implied Volatility represents the expectation of the volatility. And the Forecasted volatility is forecasting the volatility.
This forecasting is based on expectation right ? So what is the difference. Please help me understand the difference between these two.
Implied volatility = Volatility thats panning out in the market right now
Forecasted volatility = Volatility you expect
So right now IV can be 20%, but lets say in 2 days there is a RBI meet and hence you forecast volatility can be higher, say 30%.
Since the effect of Vega is highest on OTM options, can we say that for the ITM options, the effect of Delta (0.5 or more) cancels out the effect of Vega? Can we calculate up to what extent Delta will cancel out Vega for say an ITM with 0.8 delta?
Ah no, not really.
Realized volatility matters especially if you want to compare today’s implied volatility with respect to the historical implied volatility. We will explore this angle in detail when we take up “Option Trading Strategies”.
Sir, Can you tell me under which option strategy we have discussed about Realized volatility. I read Straddle, Strangle and Iron Condor strategy but didn\’t see any topic related to Realized volatility there.
Whenever we have compared current volatility with historical (in trading strategies), this is what I mean. Do check this – https://www.youtube.com/watch?v=0CnHdzTE66s&t=1s
India VIX indicates the investor’s perception of the market’s volatility in the near term (next 30 calendar days)
India VIX is denoted as an annualized percentage
Sir, Suppose India VIX valued @ 20. Now, this value is annualized% or next 30 days Volatility. Honestly I didn\’t understand above statement.
So that would be 20% for the year. In finance, most of these %s are annualized, unless specified. Like the interest rate, it is for the year, unless specified.
Lots of thanks, Sir.
Happy learning!
I have been watching markets closely and INDIA VIX seems to be fairly good indicator to point at the direction of the index,Nifty. If INDIA VIX BELOW OR AROUND 20, Nifty is not steep in up or down movements. If IV is above 20 and increasing,Nifty drops down.
The question is while choosing a spread strategy;
1- how to decide IV is low or high?
2- how to know whether volatility is going to increase or decrease?
3- can I look at BB indicator to have a guess?
4- suppose all other indicators point out that Nifty is oversold and INDIA VIX IS below or around 20, can I conclude that Nifty is going up and volatility is not going to create problem?
Many more questions to come as I continue to read.
Regards. Ashutosh.
1) One easy way to compare is to look at historical volatility and compare
2) Same as above
3) Yes, that is a good option. Plot it on Nifty 50
4) Maybe, but you should test your assumption and see how it has performed in the past.
Thanks Sir. Things now little obscured now.
I can try and help if you have queries.
For example – if the option has a vega of 0.15, then for each % change in volatility, the option will gain or lose 0.15 in its theoretical value.
The current vega for Nifty strikes is 17.So the option will loose or gain 17 in theoretical value?
Yes, that\’s what Vega indicates. But remember, there are multiple strikes acting on the premium simultaneously.
In fact this is exactly what goes on when volatility increases (or is expected to increase) – option writers start fearing that they could be caught writing options that can potentially transition to ‘in the money’. But nonetheless, fear too can be overcome for a price, hence option writers expect higher premiums for writing options, and therefore the premiums of call and put options go up when volatility is expected to increase.
If they are writing options,how could the premiums of call and put options go up? They should come down or my concept not yet clear? Pl correct me.
When you write an option, there is someone else buying the options right? Option writers want a higher price, if the buyer agrees to buy, the price increases.
If all option writers start fearing the volatility, then what would compel them to write options? Clearly, a higher premium amount would. Therefore instead of Rs.20, if the premium was 30 or 40, you may just think about writing the option I suppose.
Increasing volatility may still push the premiums even higher? Or not? And the premiums may not expire worthless. Yes, time value depreciates and that could be a chance to win the trade as an options writer.
Please two bits from you!
That right, Ashutosh. Increase in volatility increases premium and that works as an incentive for option writers to write options.
Hey there sir,
Can you please explain when a strike is near expiry the volatility is high but vega is low near expiry
Harshil, I guess it\’s explained in the chapter itself. That\’s the behavior of greeks 🙂
I think you didn\’t got my question let me explain it again the implied volatility for a particular strike is say 35%, then is this it\’s daily volatility or it\’s current month for 30 dys which we need to convert it by sd*sqrt of 30 to get the daily implied volatility for that strike.
Also in case of India VIX as it represents the volatility of 30dys meaning it\’s for 30dys whole say it is 60% , meaning it\’s the implied volatility for the whole 30 days which we need not to convert in daily volatility. Right?
Guide for the same
Thanks in advance!
Paras, so the volatility that you see is annualized. If a stock\’s IV is 35%, then its 35% per year. If you want to get daily, then you have to divide this by Sqrt of time (not multiply).
So is with India Vix, it\’s annualized.
About the Implied volatility of a particular strike is it for the period of 30 days for current month and 60 days for far month or is it the annual volatility which we need to convert into daily volatility??
Same regarding the index IND vix??
Thanks in advance!
It\’s the daily volatility that you need to convert to whatever time period you wish.
So we can say that volatility also depends on current market factors and to set up a profitable trade we need a good combination of Vega+ current market trend which is as you said as market falls volatility increases which means it would be better to be a buyer of put option as market view+Vega+Theta will favour me to earn profit on large scale due to change in premium.
Also can we say that when market rises the volatility decreases??
Yes Paras, thats right 🙂
Hi, is it true for the opposite case? when there is high volatility and the market goes up? will the OTM premium be changing with the same rate?
Premium will certainly change. But to what extent is something that depends on many other variables.
As explained as volatility increases there is a chance that market can go in any direction from the current position either bearish or bullish , whatever the case may be the buyer will be benefited as there is a chance that a particular strike will get converted to ITM.
However if as a call option buyer my view is bullish but the mark falls then in that case will the Premium\’s first change for both call and put option buyers and will further react to the market situation\’s?
That is when we say the premium will increase irrespective of call or put does that mean we have to capitalize only during the first phase when volatility is in our favour and in the next phase whatever the current market position maybe bearish or bullish it is not our concern as we have already done and dusted our trade in phase-1.
Please guide for the same.
Thanks in advance!!
Generally, volatility increases when the market falls. With the market fall the CE premium reduces but of course the increase in volatility also helps hold up the CE premium a bit.
Why will call option writers panics if volatility increases? when the contract closes in the money for them.
Because with increase in volatility, option premium too increases.
Dear Karthik,
Ahh,hmm, sry for the big query, but you are the right person to help me 🙂
I\’ve the below query
Basically my query is when the markets falls due to sudden positive(India VIX shoots up) and negative news(India VIx shoots up)
In both cases what would happen to the premium with respect to the moneyness of option
Consider other parameters are constant
Let assume market up and down based on some events
1.If the market is crashed(market fall) , hence the India VIX would increased, so the option premium would change based on the moneyness of the option
In Case of CE:
==============
->the call options the premium would have decreased its value (ITM and ATM have more impact) ->because of the rate of change of delta
->But the OTM have the low delta but because of Vega, the premium is increased
In Case of PE:
==============
->the put options premium would have increased(ITM and ATM have more impact)
->But the OTM premium also would have increased not as much as ITM and ATM (Vega effect)
2.If the market is up based on some positive news/event, then India VIX would increased
In Case of CE:
————–
– CE premiums would have increased it value(ITM and ATM have more impact)
– But the OTM have also increased(Vega) but not as much as the ITM and ATM because of the rate of change of delta
In Case of PE:
————–
-PE premiums would have decreased(ITM and ATM have more impact)
-But the OTM would have increased because of Vega (rate of change of delta is low)
Please correct my above understanding is correct or not in all cases
Its like this –
1) VIX increases, market crashes.
2) With the increase in VIX, premiums of both CE and PE increases
3) PE is also supported by delta, so put premiums increase
4) Delta works against the CE, hence CE tends to lose value, unless the VIX spike is so much that it can compensate the fall in delta
Thank you for your reply!
I just looked at chapters in that module. Although I couldn\’t understand much out of it but I came to know that these are flooded with statistics and mathematics. So can I say this module is primer to quantitative analysis?
Thats right, think of it as the tip of the ice berg 🙂
Somewhere in previous chapters you mentioned about Quants. I just looked about it on internet and found that it is really a deep thing. Does learning those things (Calculus, differentials and so on for financial markets purpose) helps in generating more neat insight into markets?
I want to know how Quants(People who actually know mathematical models for finance) are different from normal people like me(Who just know 2/3 statistical concepts)?
Check this – https://zerodha.com/varsity/module/trading-systems/
Yes! Thanks for making it more perfect
Thank you so much!
Sure, happy learning!
Yes! But if I want to trade BTST and if I am choosing deep OTM then delta will be small and directional movement will not have much effect on premium. Is this right thought process?
Also, for sake of volatility what do I have to look for? IV of that particular strike (which is deep OTM) or IV of ATM strike or India VIX?
Yes, but BTST or overnight positions, the only problem is gaps up or down opening. For IV, look at the ATM strikes IV.
When volatility increases, option prices increases attributable to vega. So here is one thought, please let me know can I implement it-
– When more time left to expiry, theta will be less for options. Also vega will be considerably high relative to theta.
– Far OTM options also will have low delta. So when market falls there will be very low effect attributable to delta but at the same time, if volatility increases, then option prices will increase.
To conclude, If I am thinking that current volatility is cooled off(on Friday may be or long holidays) and will lift in upcoming sessions (Mondays for sake of discussion) then I can decide to buy OTM call options with low delta and having more time to expiry.
What other factors may affect this implementation? Please add.
Yes, if you feel volatility will increase in the coming few sessions and if you think there is enough time to expiry, then you can consider buying OTMs. But in this situation, what really matters is the underlying\’s directional move. You need to have conviction about it.
Hi Karthik,
Is implied volatility value for any particular option in NSE option chain or in sensibull is for 30 days?
So if we have to calculate the voltility of that option for today, do we have to apply
Iv value for daily = iv value for 30 day / squareroot(30).
Ankush, not sure if I understand your query completely. You can consider the daily IV to get a sense of how volatile the stock is.
Ohh! it means whatever the final premium, it will be due to effect of multiple Greeks but we can\’t really separately tell that which Greek contributed by how much right?
Thats right.
Vega measures increase or decrease in premium for 1% respective change in volatility. So let say
Nifty spot 16600
ATM CALL 16600 has premium of 40
16600 CE Vega = 0.95
16600 CE delta = 0.47
If I expect volatility to increase(as measured by India VIX) by end of day by 6% and Nifty to move to 16700 by EOD then
from delta perspective:
delta of 0.47 translates to 47 points when Nifty moves up by 100 points
so final premium will be 40+ 47 = 87
from volatility perspective:
If volatility shoots up by 6% then premium will go up by 6*0.95=5.7
so final premium value will be 40+ 5.7= 45.7
so what should be actual final premium? 87 or 45.7 or will it be 40+47+5.7=92.7
Yes, provided there is ample time to expiry as well. Remember, the premium of an option is a function of multiple factors acting simultaneously on the option. Some tend to pull the premium up and some tend to pull the premium down.
In the tail end of Section 19.2, the following is stated
\”For example – if the option has a vega of 0.15, then for each % change in volatility, the option will gain or lose 0.15 in its theoretical value.\”
There is ambiguity in the statement. Since vega being represented as one point change, option value change should also be in points. In the above case, option value will be experiencing 0.15% change (Without the %, it may be construed as INR 0.15 for example).
Isn\’t it?
Let me recheck this, Kishore. I have a feeling I may have made another inadvertent error here. Need to re check the content once.
Hi Karthik,
How can we check if volatility of specific option is high or low. What we see in the NSE option chain is IV at that instant. Based on that single number it\’s difficult to say whether it\’s high or low. Are there set benchmarks eg: >30 is considered high and so on?
Thanks!
One quick way is to cross-check the historical volatility of the stock and the intrinsic volatility and get a sense of the current volatility.
Hey Kartik, in the above-mentioned paragraphs, the 6th point from the NSE whitepaper, you have mentioned that with an increase in volatility, the nifty can head towards any direction which contradicts what is written on Investopedia about the volatility. I also feel with volatility rising, the index might fall and therefore make sense to call it a FEAR INDEX.
Please correct me if wrong.
there was option to trade it earlier ?? as I found a blog by Nithin Kamath on z-connect dated February 13, 2014 and also found a pdf related to derivative of vix on Nse website, then if it was tradable earlier why it was stopped from trading
I don\’t think there was enough trading volume for NSE to continue the contract.
can we trade indiaVIX
Nope.
Hello Karthik,
Can we not compare the Annualized historical volatility and Vix india to take a trade in options by checking the IV of each strike and wait for the volatility to cool off in order to analyze in a efficient manner?
Yup, you can to get a rough estimate.
Hey Karthik,
I have been started practicing model based approach to trading. I want to get my hands on a book that can help me understand all the maths related to trading. Can you suggest one. thanks
Suraj, I think there is a book called quantitative techniques to markets, I forget the author\’s name though. Maybe you should check that.
THANKS SIR,
Hi,
Does VEGA affect intraday trades ?
Is it negligible for intraday ?
IF NOT THEN
How should it be used to measure risk for intraday ?
?????????????????????? ??????
Vega has an impact on intrday trades, especially when the rate of change of volatility is high. Its best if you keep track of volatility and take a call.
sir can u please answer Mr. Ajay\’s question on 13 may 2021 based on volatility. I seem to have the same doubt while going through the chapter. Basically my question is, what does the value of implied volatility in percentage represents for different strike prices.
My second question is what does the percentage increase in india vix represents? Does it represent the increase in volatility in nifty only.
thanks
Pranay, each strike has different IVs, which represents the riskiness of the strike as per the market participants. The higher the IV, the higher is the risk for that strike.
Hi,
I checked bid – Ask spread , BID = 5.20 and Ask = 7.35 , Spread – 2.15 ,
Premium closed at 5.95 and gain of +1.35
I understand greek Will increase or decrease the Premium of a particular Strike but I don\’t understand how spread can increase The Premium .
Can I Take These assumptions on this 472 Strike
1. Volume and OI of this strike Was in
thousands where as Strike 482 OI and Volume Was in lakhs.
2. when spot increase , initially buyer increase The Demand of the Contract and it tends to increase in premium as We can see this strike is illiquid in Term of OI and Volume as compare to Other strike . So, neither buyer increase The Price Nor seller decrease The Ask Price and Premium Stuck with a Positive Sign as There is Major Difference in between BID and Ask spread.
I am Confused , May be You Get Point and correct me
Imagine I place a market order in an illiquid option, what do you think will happen? Order will get executed at odd price and premium increases right?
Karthik Sir,
All The OTM strikes which are gaining Yesterday are losing premium today strikes like 472 and 482
and All the other strikes gaining Premium Today which were Losing Yesterday.
Still My Question is why Strike 472 only gained Premium Yesterday
Again, it\’s the closest to the ATM strike, hence slightly better liquidity I guess. Did you observe the bid-ask spreads also?
Hi Karthik Sir ,
I Ask you Lot of Questions . Hope i don\’t Bother you .
Yesterday, I Was watching BPCL Option Chain and observed some Premiums movement . I Hope I can share a screenshot .
I Will Write horizontally each Strike and Their delta Values ..
Spot Closed – 447.50 , Expiry – 28-Oct
Strike CE – 472 , 475 ,477 , 480 , 482
Delta – 0.29 ,0.34,0.35,0.23,0.22
Gamma – 0.0086, 0.0065, 0.0059,
0.0076, 0.0073
Theta – -0.31, -0.48, -0.53,
-0.27,-0.27
Vega – 0.38, 0.4, 0.41, 0.33, 0.3 Premium- 5.95, 12.15, 13.85, 4.80,4.85
Change in premium= +1.35, 0, 0, -0.15,
+0.10
Implied Volatility – 32.18, 51.78, 57.45,
34.41, 36.81
OI = 66,000, X, X, 4,66,200,
1,26,000
Volume – 63,000, X, X, 12,43,800,
46,800
Points to be noted…
1. Why we saw a Positive Response
only in Strike 472, 482 , There is
massive positive Change in Strike 472
only. If we Notice that low delta is the
factor that prevent to Fall in Premium
then Strike 482 has Low delta Than.
Strike 472 ,still 472 has gain More in.
Premium .
2. If we Check IV factor still Strike 482
has more volatile which Help to Rise
the Premium but still 472 Strike gain.
More Change in Premium.
3. Strike 480 also has Low delta but
still it\’s Premium Fall and Have more
IV Than Strike 472.
4. If we consider Volume and OI still
Strike 472 hasn\’t won from Strike
482 and 480.
5. My Question is Why only Strike 472
gain in Premium
When you consider greek, there is an underlying assumption that all options are liquid. If the options aren\’t liquid, what really matters the most is the demand-supply situation of options and their respective strike. Given that, I\’m guessing that the strike under consideration is not very liquid here, hence the greeks don\’t really obey as expected :).
For buying a call option shd i check IV… what shd be IV value for in the money call option
Ravi, IVs can vary between indices and stock, there is no common IV structure across markets.
Hi,
Is there any plan to teach about GARCH.
Nope, I don\’t think I can do that. Also, it may be way too complicated to explain.
You can\’t say, all strikes above ATM are ITM and Deep ITM. it\’s true only for Put options. For call options, All strikes below ATM are ITM and Deep ITM.
Of course 🙂
I think it needs some clarification. Let me know if I am wrong somewhere. Correction is suggested in bracket.
On 24th August, Nifty declined by 490 points, so all call options which had ‘noticeable Delta’ (like 0.2, 0.3, 0.6 etc) declined. Typically ‘in the money’ (I think it should be said ITM and slightly OTM options instead of saying just ITM options. Because before decline of 500 points Nifty would have been 8300. So, upto 8600 all strikes will be slightly OTM, not ITM. Also, slight OTM has some noticable Delta, while deep OTM has very low delta. So more than 8600 strike prices are deep OTM and that\’s why they could not show movement in line with underlying.)options (as on 24th Aug, all strike below 8600) tend to have noticeable Delta, therefore all their premiums declined with the decline in the underlying.
‘Out of the money’ (Deep OTM to be specific) options usually have a very low delta like 0.1 or lower. This means, irrespective of the move in the underlying the moment in the option premium will be very restrictive. As on August 24th, all options above 8600 were ‘out of the money’ (Deep OTM to be specific) options with low delta values. Hence irrespective of the massive fall in the market, these call options did not lose much premium value.
Rajnish, there are two ways to look at this – either from the strike price perspective or simply from the delta perspective. ALL strikes above ATM should have higher deltas (higher than 0.5), hence ITM and deep ITM. All strikes below ATM will be OTM or deep OTM. Delta above say 0.3 and the associated strikes will react more quickly to price change compared to the lower delta strikes.
Hello Sir,
Why IV of options strike price increases ( especially of PE ) near expiry even if there is no big movement on either side.
Thank u
Cant really answer why volatility increases, Abdul 🙂
Hey Karthik!
So nice to see someone consistently providing such quality content and support over a good 6 years !!
Eager to apply the learnings and excited for the subsequent learnings that come up as well!!!
Also, regarding the August 24, 2015 incident (shared in the chapter), are such occurrings not the norm wherein the OTM option prices shoot up (due to low delta and high vega effects) whenever there is a considerably huge crash in the market?
These things can happen in the market, Mahavir. Quite common these days 🙂
Do really one can make enough money in trading to live a desirable life?
Depends on your expectations out of desirable life. But its easier said than done 🙂
Do trading is a good carrer option?
It is not viable for most people, Sam.
Great work, kartik!
One of the best explanations of option greeks
I suggest you to bring a module for quantitative analysis.
Super curious to learn, but can\’t find the best like you 😉
Thanks, btw you can check this module – https://zerodha.com/varsity/module/trading-systems/
1) Delta: High for ITM
2) Theta: High for OTM
3) Gamma: High for ATM
4) Vega: High for OTM
Correct sir?
thank u
All these things really depends on the market conditions. Very hard to genralise.
Dear sir, I have read the chapters of volatility 3-4 times but I can\’t seem to have an understanding what volatility really is.
Volatility is the riskiness as you say.
But what riskiness?
For example nifty spot is 14,700.
In OI chain, IV of 15,000 CE is 14.78.
So what is this 14.78?
a) Is it that there is 14.78% chance that nifty will reach 15,000?
b) Is it that nifty may go up and down 14.78% from its spot i.e. 14,700?
c) Is it that nifty may go up and down 14.78% from the strike 15,000 (as this 14.78 IV is of strike 15,000 CE)?
d) Or is it something else? If so, kindly explain.
Ajay, think about the volatility figure as a risk indicator. The higher the risk, the higher is the value of the indicator. Also, think of the figure as the % points it can go against your position.
Sir when volatility shoots up premium will increase
On other side we can write the option and collect premium and exit when volatility cools off
Assume there is good news and markets are expected move higher in such a case option will be expiring ITM if I sold OTM strike clearly I would be losing money right
Dear Sir, I understood the overall concept of volatility and implied volatility.
But what does implied volatility on a particular strike price mean? I am unable to understand the that. Kindly answer my query.
Thank you.
It is the volatility of a particular strike.
Similar analysis of Vega and volatility requested for 23/03/2020 please
What is the possibility of losing entire capital in option selling if leverage is not taken,comments please
It depends on the strike you choose, Mohit.
I am really surprised no one has developed it yet, considering there are so many indicators for almost anything else.
Hmm, I\’m sure there is something available, its just that I don\’t know 🙂
Hi Karthik,
Great content on volatility.
Do you know any universal technical indicators that can be used to identify high volatility vs low volatility?
I know about indicators like ATR, Bollinger Bands etc. These are a bit subjective. For example ATR can be 40 in stock A meaning high volatility, and for stock B with 40 it can mean low volatility.
By universal I mean, the same value means the same thing for all stocks. For example, MACD line above 0 (say 5), means the instrument(stock, commodity, whatever)..is no longer in downtrend.
The VIX comes close but unfortunately it applies only for broad based indices.
Jay, no, I\’m not aware of one, although I think this could be easy to develop 🙂
Why is the vega higher for an OTM option? I do understand that it is higher for an option with longer expiry.
Hmm, not really. Can you please share the context?
Yes I do understand what you say is common-sensical. But your previous chapter takeaway says \”Higher the SD, higher is the range, and lower is the premium collected\”. Then as per this logic, the premium is supposed to be low for a higher volatility (higher SD) (theoritically speaking) right?
Ah, sorry. I got the context, yes higher the SD, higher is the range, which means that these are OTM (or deep OTM) options which has a lower premium.
Hi,
There\’s one doubt. Lets take Nifty for example. You say that the option premium rises as the volatility rises. But, we know volatility is nothing but SD of the underlying price. And as the SD increases, the possible range of Nifty increases, which means that the options premium should come down right? Is it because the option seller expects a higher premium to take up more risk since the volatility is high? This is a doubt coming out of theoritical explanation.
Hmm, not really. The higher the volatility (or SD), the higher is the chance of the option expiring ITM, which is not good for the sellers. Hence the sellers expect higher premium.
We do make Delta zero / Neutral strategy on same note can we make Vega zero strategy ?
Not possible to eliminate Vega and make it neutral. Or at least, I\’m not aware of. Need to figure this out.
Hey karthik you have explained options in very simple and alluring way. My query was what is the role of open interest(OI) in options.
OI and Price should be looked at through the same lens. Do check this – https://zerodha.com/varsity/chapter/max-pain-pcr-ratio/
Your method of explanation is the best in world!
Happy reading, Dinesh!
I have the following doubt related to the increase in CE premium by 50-80% for OTM strikes even with the market crashing 5.92%,
You have attributed this due to an increase in Volatility, as due to sudden up or down movement IV increases theoretically for both CE & PE, I got that.
– But practically for OTM CE strikes premiums to increase, ideally, there should be more buyers & fewer sellers, but when you already see the market falling who would logically turn out to be OTM CE buyer? I suspect very few & rather there would be more CE sellers would be present.
– Premiums are bound to increase as it was set by formula? or Practically there need to be some buyers who are willing to buy the CE option when market is falling?
1) Different opinions is what makes the market and also this is the reason why liquidity is important. As long as there is liquidity, there will be buyers and sellers in the market
2) It is a function of greeks and supply-demand as well.
I found this for historic daily/weekly/monthly/years returns for all indian indices.
https://www.bloombergquint.com/markets/equities/historical-returns
Is this good?
Yes, it is.
Dear Karthik Sir,
Just a follow up to my earlier question.
So when I am calculating a fair value price of a future expiry Feb 25
There are 17 days to expiry but there are 13 thirteen trading days.
Both calculations results will be different.
What should I do?
As I mentioned, NSE would consider 17, I\’d consider 13. I\’d suggest you use both for a while and see which one works better for you.
Dear Sir,
Where can I find the daily average and the annualized average for a certain stock/index.
On the nse website it only shows the daily and annualized volatility for futures?
Jacob, I guess you will have to calculate this, it is quite easy on excel.
Hello Sir,
When you calculate volatility for the period of next X days (for writing options/maintaining a stop loss) do you count non trading days?
For your annual volatility calculation you use 252 day.
For monthly it should be 21 and weekly 5 days correct?
But when used for fair price calculation of futures/ B&S they use non trading days as well. Does that not cause problems in the calculation?
I tend to look at only the trading days and ignore the non-trading days.
Dear Sir,
IV for ATM options are the lowest while the Vega is the largest for ATM options.
Can you explain this if possible, not understanding it properly?
Have discussed this cross many comments, Janta. Request you to please check the comments section.
Is there any particular relation of Vega with respect to moneyness of the option?
It was mentioned that OTM has high Vega but what about ATM, ITM and deep ITM?
Yes, Vega has an impact on the moneyness of the option. With the increase/decrease in vega, the premium too increases/decreases, hence the change in moneyness of the option.
small update: link of the white paper on IndiaVix is moved to https://www1.nseindia.com/content/indices/white_paper_IndiaVIX.pdf
You might want to update here.
You have done a great job! Thank you!
Thanks, I\’ve not looked it the change in link, but I\’m sure this will help 🙂
And iv is monthly or daily basis.
Thank you.
Usually expressed on an annualized basis.
What is the difference between volatality we see on equity derivatives on nse website and IV on options chai . I saw for indusindbk share, it was 4.7 on equity derivatives and 75 on option chai .
Please explain
Thank you.
Website is the volatility of the stock on an annualised basis, IV is the strike\’s implied volatility.
” MAYBE it’s a good idea to write options and collect the premiums at the start of the series when the premium is high” This is for the risk-takers right?
Depends on how you evaluate risk. While risk takers would right say 3SD away, risk-takers may want to write 1.5SD away.
Hello Kartik, How can one benefit from the IV data present in option chain?
Do check the chapter on Volatility, have explained this in detail across a couple of chapterss
Is it true that India vix and indexes are inversely proportional to each other?
Generally speaking, as ViX goes up, Index falls.
Hey Kartik, could you explain the part where you said, \” MAYBE it\’s a good idea to write options and collect the premiums at the start of the series when the premium is high\”
That\’s because, at the start of the series, the premium is high owing to a lot of time factor. If you identify the right strike, it makes sense to write it and collect the premium associated with it.
Sir can you make on step by step process on option writing with example
sir , when we do a spread ( like bull call or bear put ) it shows that increase or decrease in implied volatility effects the P&L (though underlying wasn\’t very bullish or bearish) is it true .
thank you .
Thats right, volatility has an impact on the P&L.
One more thing sir, if i have a stronger conviction then i can go ahead and short a ITM strike through selling CE. And so I as a call option seller should fear getting into deep ITM, right? And hope for reaching to ATM or OTM as soon as possible, is that correct?
Am extremely sorry sir for asking such inane questions to you, but getting a little muddled, as studying all these things for the first time. I hope you will understand and not mind responding to my curious queries.
Many thanks as always!
That\’s correct, Aarti. As a seller, your best outcome is when the ITM CE you\’ve shorted transitions to OTM. And don\’t worry about asking questions, ask as many as you want, till everything is cleared up for you 🙂
Sir I was looking at Bank nifty and at a strike of 26500, the premium is 28.3Rs for CE in NSE option chain. Now if i plan to sell call option then premium receivable should be= 25×28.3=707.5Rs for 1 lot of bank nifty. But as per the Zerodha\’s F&O margin calculator, the premium receivable is being shown as 916Rs at the very same time. So why such a vast difference is arising?
Your response would be much appreciated!
Guessing the premium has increased. Data on NSE website is delayed, the data on the terminal is real-time.
Hi Karthik,
When you say IV was not the highest, what are you comparing it against?
I was talking about IV at ATM with respect to ITM and OTM, but I got the answer in the next chapter (\’Volatility Smile’ :)).
As you quoted in the next chapter theoretically IV should be the same for all stricks but in reality, it forms \’Volatility smile\’.
why is that?
Thanks
-Ganesh
That\’s because of the demand-supply mismatch.
Hi Karthik,
can you ask your IT team to make this comment text box as rich-text type so we can attach the images, pdfs, or text styling?
it will help readers to frame there question better.
Loving Varsity
Thanks
-Ganesh
Will do. For images, you can upload on the drive and share the link here.
Hi Karthik,
while reading this chapter I looked at the nifty option chain at https://web.sensibull.com/option-chain?expiry=2020-10-29&tradingsymbol=NIFTY.
Vega (The Vega of an option measures the rate of change of option’s value (premium) with every percentage change in volatility.) of ATM was the highest with making normal distribution pattern (decreased going toward OTM and ITM ), but the IV was not making any pattern (IV is not highest at ATM).
are Delta, Gamma, and Market direction affecting IV? and how? can I conclude anything based on IV and Vega?
Loving Varsity
-Ganesh
When you ay IV was not the highest, what are you comparing it against?
Thank you Karthik ! Your response( and that too so quick) is really of great help. :).
Happy reading 🙂
In sensibull, intraday IV is not available . Only day wise is available . During intraday option trading, i am referring to India Vix to judge whether the option premium pricing is on higher side. Hope the thought process is correct.
Yes, India ViX can be considered as a proxy for IV.
Hi Karhtik,
What is the relation between IndiaVIX and IV of Bank Nifty? I have observed that during intraday trading, option prices are fluctuating due to volatility.
I have seen IndiaVIX fluctuating. Where can we observe real time for IV of Bank Nifty ?
India ViX is a proxy for the market\’s implied volatility. Not sure if real-time IV is available anywhere, maybe you should check Sensibull.
The white paper link can be updated here as it shows page not found.
Let me check on this.
hi kartik, so can we just take india vix and convert it into 1 day for getting range in nifty while trading intraday?
Yes, you can by do that. Make sure you divide the ViX data by sqrt of time.
Happy Teachers Day, sir.
I get to learn lot of things from you and every minute spent in Varsity is amazing. You inspire us. God bless you with happyness and wealth
Thanks for the kind words, Vaishakh! Happy learning 🙂
\”\’With increase in volatility, the Vega of an option increases (irrespective of calls and puts), and with increase in Vega, the option premium tends to increase. On 24th August the volatility of Indian markets shot up by 64%. This increase in volatility was totally unexpected by the market participants. With the increase in volatility, the Vega of all options increases, thereby their respective premiums also increased. The effect of Vega is particularly high for ‘Out of the money’ options. So on one hand the low delta value of ‘out of the money’ call options prevented the option premiums from declining while on the other hand, high Vega value increased the option premium for these out of the money options.\’\’
Dear Karthik,
what does this means in perspective of market participants… i mean its just the delta and vega that played a role or more of call writing has change the premium..?
i am totally newbie, so parden my silly query..
sandip maru
Thats right, in this case the dominant factor was the delta and vega.
hello karthik
how do we come to know that for a particular strike price the iv given on option chain is on higher side or lower side.
YOu will have to compare it with the underlying\’s historical volatility, this will give you a rough estimate.
sir ,
please do explain this in detail \”1) You can calculate the historical vol, compare it to current IV and get a sense of how the current volatility is placed.\”
thank you .
Meaning, you can see what the historical volatility for the underlying has been (easy to calculate on excel), compare it with today\’s IV and that should give you a rough perspective of where the volatility is. For example, if for a stock, historical vol is 22% and ViX is 35%, then I know today\’s volatility is high. However, you need to remember that this is only a rough estimate.
while trading in options do we have to pay 20rs ( brokerage charges) for both buying and selling or 20rs for buying and again 20rs for squaring off (selling).
It is 20 per executed trade.
sir,
Does zerodha charge on converting nrml order to mis and again on end of trading session mis order to nrml ?
thank you.
No charges for position conversion.
Hi Karthik,
1) How is the Implied volatility calculated? Can we learn how to calculate that, or is it just a bit too complex ?
2) Well if it is hard to calculate IV, lets say I use the IVs given in Sensibull or in NSE date and compare it with historical volatility that you\’ve taught in one of the modules.. how do I make an apple to apple comparison? The IVs given in NSE are annualized,right? and they are the deviation from which mean exactly? the mean of the whole year\’s return? Let\’s say I have to compare last 10 days to expiry historic volatility to IV.. how do I go about doing it?
Thanks
1) Its quite complicated math 🙂
2) YOu cant, hence it is considered \’quick and dirty\’ comparison
3) Yes, these are all annual numbers. If you are looking at 10-year historical vol number, then you will have to scale it down to yearly by dividing the 10 year number by square root of time i.e. 365 days.
thank you sir .
Good luck!
Sir ,
1.Like you said in nifty option IV 20 or below is low IV and 30 or above is high in IV .how can we know highs and lows for any other stocks or other indices like bank nifty.
2. when we put a MIS ,BO and CO orders we get to sell options for not more 20,000 . now due to new SEBI rules do we need to pay whole margin amount like 1,40,000 to do options writing .please explain .
thank you .
1) You can calculate the historical vol, compare it to current IV and get a sense of how the current volatility is placed
2) Yes, complete upfront margin i.e. SPAN+Exposure is required
Vega increases alright but why did the CE premium increase. If premium increase that means someone is willing to buy the option at that price, why would someone buy a CE at a high price when the spot is falling drastically. Shouldn\’t the PE option premium increase at such situations?
why would someone buy a CE at a high price —- > because they expect it to reverse and go higher. Remember, different opinions are what makes the market 🙂
hello sir ,
In this chapter we learned that with increase in volatility premium also increases.
sir which volatility are we talking of ? historic or implied ?
Implied volatility is what we are referring to.
hello sir,
sir the stated example above , with increase in volatility the price of OTM CE shot up albeit the underlying price falls.
Is this once a blue moon thing or a general phenomena ?
No, this happens whenever there is an increase in volatility, which can happen every now and them.
Sir
Is it true that SEBI is removing leverage from 1st of December.
Yes, you can check this post – https://tradingqna.com/t/no-additional-intraday-leverages-from-aug-2021-in-indian-capital-markets/84561/187
why VEGA affects OTM CE more in terms of premium ???
Vega has the same effect on both CE and PE, do partiality to CE only 🙂
why does VEGA affects OTM options more in terms of premium? as you said in last case study
Higher the time to expiry, higher is the chance for the option to swing either way. Hence vega has a higher effect.
Similarly, when volatility increases, the stock/index price starts swinging heavily. To put this in perspective, imagine a stock is trading at Rs.100, with increase in volatility, the stock can start moving anywhere between 90 and 110. So when the stock hits 90, all PUT option writers start sweating as the Put options now stand a good chance of expiring in the money. Similarly, when the stock hits 110, all CALL option writers would start panicking as all the Call options now stand a good chance of expiring in the money.
How did you say that Put and Call options have good chance to expire ITM??? Please elaborate.
With increased volatility, the chance of the stock moving all over the place increases, this means there is an increased chance for both CE and PE to expire ITM. This is what I mean by that 🙂
Sir,
I am not able to understand why we have different IVs for same underlying asset, same expiry, same events( May be Corp Actions, RBI policies OR else)..
kindly explain why do we have different IVs?
IV is different for different strikes. Each strike is a different option, hence different parameters work differently on these strikes, therefore different IV.
hi karthik
suppose i m bullish on stock like sbi in 5 days time in second half of month .
i have created ratio spread
buy ce 180
sold ce 200
max profit 50000 suppose
ideally at wat profit percentage we should close are position .
Ankit, ideally it should be 100% upon expiry. But if you want to exit before that, then that depends on your risk and reward expectations and the associated market condition.
hi karthik which one to buy .
1. https://www.amazon.in/Option-Volatility-Pricing-Strategies-Techniques/dp/155738486X
2.https://www.amazon.in/Option-Volatility-Pricing-Strategies-Techniques/dp/0071818774/ref=dp_ob_image_bk
First one. I\’ve not read the 2nd one.
hi karthik.
can u suggest best books on
1.options selling
2.hedging of option selling like big players and big institutions do .
For both, I\’d suggest – Option pricing and volatility by Sheldon Natenberg.
hi karthik .
i want to know when big players or big institution make their position in nifty and bank nifty options whether selling or buying option (weekly option ) .so it can reflect in open interest and change in open interest .
is it on friday .monday .tuesday wed or thursday according to ur expereince in the market .
when they take entry and exit .probality kaha zyada hoti h
No idea about that, and I don\’t think weekday matters. They set up trades based on when the opportunities come by.
hi karthik ,
have u written article on volatility skew or volatility smile .
where can i check it on real time basis
any link or source plz provide
Try sensibull.com, Ankit.
Hi sir,
Does the option premium depends only on option greeks? or does it has any relationship with demand and supply?
Why there was no IV on banknifty on 18-06-2020 (weekly expiry) for any of the strike?
The demand and supply have its own influence on the premium, Gokul, although the prime drivers are the greeks.
Hi,
So in the example above of 24th Aug 2015, understand the impact of vega keeps premium of OTM calls +ve. However these would be LTPs. My question is shouldn\’t the fall show up in the bid/ask spread. Which is more driven by live trader wanted to bid for a call of 8900 where the different in Bid ask if hardly 0.20 bps.
One would expect more like what we see in 9050CE bid/ask. Where now the bidder would have significantly lowered his bid levels to the ask levels (which would still be around the LTP lvls). Shouldn\’t that be the case. Logically why is someone still ready to purchase a call of 8900CE at such elevated levels ?
Yes, eventually all of these factor into the order book and show up on the bid-ask spread. The reason why ppl buy is they have the exact opposite reasoning of a seller, remember different opinions is what makes a market 🙂
Thanks for the update.
Happy trading!
Thank you for the update. Please throw some light on the following , Company A’s Historical volatility is at 40% so based on this premise
1. Logically then for Ex : If Comp A’s OTM CE / PE has a IV of 60 % and both has a premium of 10 Rs, does this imply that based on its IV, the premiums has a higher chance to fluctuate at 60% from current premium 10 rs (based on the underlying).
2. If all else remains same and I need to Sell ( Write) OTM PE/ CE strikes based on only based on IV than how does one interpret IV of 60 or an IV of 20 for an OTM what difference does it make.
3. Does higher IV strikes have a higher premiums ? If yes, I can choose to write only higher IV strikes at start of the expiry and wait for theta to work.
I’m tryin to interpret IV better from a different angle.
1) Yes, and also implies that the premium of 10 could be high
2) IV of 60 is high, and hence the associated premium. You need to look at selling opportunities. IV of 20 is low, and hence the associated premium. You need to look at buying opportunities.
3) Yes
Hi Karthik
is there any way we can find historical and average Implied volatility for particular stock options? I guess India Vix is for the broader market but if I am thinking of writing an option then can I find relevant historical data of implied volatility of a specific stock?
Historical IV I\’m not sure, but historical vol is easy to calculate.
Assuming the historical vol of HUL is at 62% for previous 1 year.
Is Implied volatility calculated and reflected for Spot or only for options ?
1. Current Spot is at 1975 , and annual Vol is at 62% So seeing the IV for ATM CE is around 33% and PE at 37% does this say IV is lower ?
2. Only 2400 CE and 1600 PE has a 60% IV , so is it safe to assume and write these option, as these strikes has a Sd or Vol of 60% ?
3. If I need to select strikes based on my SD or IV than would 2400Ce be the right candidate here ( just for assumption) , as historical VOL for HUL is at 60%.
Not sure if I have missed anything on this.
1) Historical is for the spot, IV is for options. Although not very clean, you can compare these two to get a perspective of the volatility
2) Not the PE, but CE maybe 🙂
3) Yup
Pulp Fiction, Kill Bill, Reservoir Dogs, Django Unchained etc
Thanks for the titles, do you have more? (Just kidding)
You are doing a wonderful job in this course, thanks from my side behalf of all readers.
Looks like you like Tarantino as well 🙂
hello sir
i want to know where i see change in volatility any site which you recommend
I wish to enquire about an aspect. As you\’ve discussed, there are 4 option greeks which decide premium value in real time.
But if we think in general, is it right to say that at the end of day, what decides derivative prices is supply-demand relation.
The reason I\’m asking this is because suppose we have some greek values in hand and rough market idea. This would lead to some inference. But with this knowledge in-hand, at ground level, what if people take a foresight and do something else. Then can we say that the option greeks and entire pricing model has failed us?? (Please answer)
Yes, demand and supply play and important role in option pricing and to some extent, that is captured in the delta of the stock.
Please Update the India VIX white paper URL to http://www1.nseindia.com/content/indices/white_paper_IndiaVIX.pdf
Current URL broken
Hey thanks so much, Rajendran. Will update the link.
Sir
The incidence of 24th Aug 2015in the Nifty options, it seems rather than the underlying, it is the Greek (Vega here) that is controlling the options premium to a large extent. In fact, the options premium of OTM are moving adversely to the direction of movement of Nifty. Does this imply that derivatives have little to do with the movement of underlying and the options Greeks become the deciding factors ?
Thanks
Yes, Vega plays a massive role in option premium pricing.
Sir
The Whitepaper mentioned for VIX no longer exists on NSE\’s website. From where now we can have calculation of VIX ?
Thanks
They must have changed the location, request you to Google the same. Thanks.
Thank you for the prompt response. Also as soon as I read the first topic ( = volatility smile ) of the next chapter, my doubt was explained …..
Thanks for such comprehensible texts!!
Good luck, Ishan!
Hello Karthik,
I am an absolute newbie who\’s trying to get his baseline set before venturing into trading
Just finished reading this chapter on vega and have a small doubt. You\’ve mentioned this example of NIFTY options 24th August 2015
To quote you
\”\” Typically ‘in the money’ options (as on 24th Aug, all strike below 8600) tend to have noticeable Delta, therefore all their premiums declined with the decline in the underlying.
‘Out of the money’ options usually have a very low delta like 0.1 or lower. This means, irrespective of the move in the underlying the moment in the option premium will be very restrictive. As on August 24th, all options above 8600 were ‘out of the money’ options with low delta values. Hence irrespective of the massive fall in the market, these call options did not lose much premium value.\”\”
Since the CNX NIFTY closed at 7809 ( after a fall of ~ 490 points) shouldn\’t all the Call options above 7800 ( or above 8299 – previous closing ) be OTM?
If yes then why the selective effect of vega on the CE options above 8650 ?? since all the OTM options tend to have a delta close to 0 including the ones between 7800 and 8600.
Thanks and apologies in advance for my doubt may stem from absolute naivity..
If Nifty closes @7809, then all call strikes above 7800 will be worthless or OTM (except for the 7800 CE, which will have a marginal value of 9). Very deep OTM reacts to changes in vega, that\’s how options behave 🙂
Hi Karthik,
You said above \”So if you are wondering why your long options are not working favorably in a highly volatile environment, make sure you look at the time to expiry\” what does it mean?
Is this because of the higher premium in a highly volatile market we should not go long on options ? or is there any other reason for this?
Time to expiry, volatility, and market direction, they all go hand in hand Tarun. They all act in their own way and exert their force on the option premium.
\”By now everyone remotely connected with the stock market would know that on 24th August 2015, the Indian markets declined close to 5.92% making it one of the worse single-day declines in the history of Indian stock markets.\” Compared to what\’s happening now, 5.92% doesn\’t look worst LOL.
Haha, you bet 🙂
Sir whitepaper link is not working. Quote the content from above.
\”You may have heard or noticed India VIX on NSE website, India VIX is the official ‘Implied Volatility’ index that one can track. India VIX is computed based on a mathematical formula, here is a whitepaper which explains how India VIX is calculated –\”
Ah, you need to check with the exchange for this. They may have moved it to another location.
Fix the link of Whitepaper.
Hi Karthik,
I have a query on a comment by Gurmukh on 16th Dec 2016.
Crux of his comment is – an OTM PE\’s premium decreased when nifty lost few points. Time to expiry isnt clear, but as per the greeks that time, premium should have been down by 11, but in reality, it got reduced by 7 points. His assessment of the situation and calculations look correct.
In response to this you point to – http://www.thehindubusinessline.com/portfolio/technically/when-options-strike/article7596687.ece. Though this link is valid, but it\’s subscription only link.
Can you share your analysis of this or similar scenario?
Regards,
-Sachin
Sachin, what it really means is that the premium depends on the volatility as well. If the volatility decreases the premium too reduces and vice versa. You can see this in the markets today….mkts is up about a per cent, but the call options are losing money.
Hello Karthik
The content provided is really well. Can you suggest some of the best books to go through for options trading?
Sheldon Natenberg\’s book on option volatility and pricing is really good.
Thank you.
One important ( for me 🙂 ) question.
How this gap up or gap down of stocks and indices (Nifty/BankNifty) are calculated? How do we know if tomorrow it will be gap up or gap down?
Also you shared Zerodha Pulse for news but this is not effective. How do we know news about particular stocks or indices where the impact is more for either increase or decrease in price
If you can share definitive info on the news would be really helpful
Gaps can be calculated based on the previous close and today\’s open. You\’ll never know when gaps will be formed, depends on the overnight news flow. You will have to track the stocks specific news to get a sense of how the gaps form.
Dear Karthik,
The following \”Double quotes\” ones in each point below seems confusing and contradicting.
Can you please clarify?
1) Implied Volatility represents the \”market participants expectation\” of volatility
2) \”India VIX represents the implied volatility\” over the next 30 days period
On 24th August the volatility of Indian markets shot up by 64%. \”This increase in volatility was totally unexpected by the market participants\”
If India VIX is the market participant\’s expectation, why on 24th August was unexpected by the market participants?
India ViX is derived from the implied volatility and therefore its a proxy for implied volatility, but not the same thing 🙂
Hi Sir,
So if volatility suddenly increases then can change in premium be more than what the old delta is saying?
Yes, a sudden spike in volatility has an impact on the premium.
thanks for the advice
The price of an option shown in kite is the output of Black & Scholes or last traded price? If last traded price then how we can know the fair price? Who determines the volatality, the big players, I mean sellers of options?
The price that you see on Kite if from the exchange, which is market-driven. So yeah, its the last traded price. Volatility, price and everything else is determined collectively by the market and not any single group of operators.
Can you provide any excel or any calculation to find realized volatility? Also volatility studies are majorly for option writers and not for buyers?
Its for both. Realized volatility is nothing but historical volatility. It can be calculated by using the \’=STDEV()\’, function in excel.
why are there bid and ask quotes in option if the premium is to be decided through a formula and not by the market participants
The fair price of the premium is derived from the Black & Scholes option pricing model. However, the premium gets traded above or below the fair price based on the demand-supply of the strike. Think of it as the real estate premium set by the government and the actual price that gets traded in the market.
Good morning Dear Karthikji,
I\’ve few questions about weekly Nifty/BankNifty options… although in varsity whatever u taught us on Nifty examples are the MONTHLY OPTIONS one as it was that only those days.
1)For weekly options how should we view volatility & theta. For eg at the beginning of 1st two weeks of months does Volatility will have effect like blue line of graph in two successive weekly options & same volatility will have red line effect at the end of last week expiry of Nifty ??
2)Or does theta & volatility is functioning different for monthly & weekly options seperately ??
3)If suppose I want to initiate Call Ratio Backspread or Bear Call ladder when volatility is low, then is it good in 1st two weeks or last week or it\’ll be same across all four weeks ??
Kindly reply all my concern query as per your wise opinion & thanking u for giving ur valuable time 🙂
1) Yes, everything remains the same. The timelines shrink.
2) No change, they function the same
3) If the position is net long, then its better to initiate in the first half, else its better to wait till the 2nd half
Just finished the volatility module. Clearly, the module was written when there used to be month long option series. In changed scenario of week long option series, how to trade using volatility strategies? Please suggest adaptations/modifications.
Pradeep, the timeline has shrunk, but the underlying philosophy stays the same.
Weekly expiry options are like T20 matches and I fear you can\’t play a T20 game with the Test match approach.
While the approach differs, the tools of the game remain the same.
Hi sir, on 23rd may, nifty moved up 125 points approx and it\’s in 11800 levels.. But the premium for 12000CE was reduced 8.5% and one telegram Grp admin stated that it is because of volatility. If volatility increases then premium should increase right? How is this possible? NIFTY moved up hence CE premium should go up buy it reduced on the same day. Not only this sir, I\’ve seen a stock moving up daily without any decline, let\’s say it\’s premium for a random strike is 200rs… the underlying is increasing after 2 days premium is 250rs.. after 5 days the underlying still moves upwards but premium comes back to 200rs again. How is this possible because effect of theta is not possible for this huge difference.
Bala, you should read these case studies – https://zerodha.com/varsity/chapter/case-studies-wrapping-it-all-up/ 🙂
Sir as you mentioned for each % of change in volatility options Vega will gain or lose in theoretical value.
Yesterday markets gained 165points so 11600pe lost 32rs in its premium value but volatility has been increased for this option.let\’s say Vega was 9.6 will this value is gain for this option premium because of increase in volatility.
The increase/decrease in premium is not just about the volatility. The delta too has an impact. Think of it as multiple forces being exerted on an object at the same time. The end result will depend on which of these forces comes out as the strongest.
Ohh I have not asked the question properly here is my exact question sir.
On Friday 31/5 spot closed at 11922.
11800pe
premium 249
Expiry 27/6
IV 8.96
Delta 0.738
Theta -3.315
Vega 9.916
Next day on Monday market had a strong upmove of 165points at the end of the day 11800pe premium was closed at 382.
165*0.738delta=121.77 lost in its premium value along with -3.315 theta decay.
IV from 8.96 increased to 9.82 so for increase in volatility Vega of this option 9.916 will it get gained for this option premium.
165*0.738 = 121
121+249 = 414
I guess, you are concerned about the actual premium value which is 382 and not 414. This deficit is attributable to both time value and the reduction in volatility.
Sir but here the volatility of 11800ce has increased from 8.96 to 9.82 with increase in voltality and strong up move in index of 165 points both delta and Vega Should take the premium higher than what it is at 382.
Can you double check this? Because when the price increases, the volatility usually drops.
sir, I have to finish 4 more remaining chapters but can\’t wait to appreciate the fact that u have explained the topics with such clarity and even while recalling the topics from previous chapters u go on to write almost a paragraph to remind the topics, again and again, thanks for putting in this much effort for writing the material
Thanks for the kind words, Sahil! This feels so good to hear, happy learning 🙂
Dear Karthikji Post elections results although nifty IV falls to normal for call options around value of 11 for 1-2 strikes from spot but Put options had huge unequal values. The IV of puts 1-2 or even 3 strikes from Nifty spot is having IV values 16 to 17 for weekly options & same for monthly too. Although due to recent high volatility the annualised volatility is now at approx 22.5. I saw this in nse sites. 1)Why this huge imbalance between puts and calls ??
2)What this indicates & can one sell put options with some OTM hedge in hope of volatility getting cool down ??
3)NSE websites calculates IV & options price etc on 10% interest rates(written on bottom) whilst RBI 91 days interest rates as u\’d recommended us is little above 6%. Which one to refer then ?? Will it makes any difference
Thanking you for giving your kind time 🙂
1) The IV of PE and CE is never the same (although it is intuitive to think so) because of the underlying street sentiment and therefore the demand-supply situation for each strike
2) Yup, if you think the volatility will cool off, then it does make sense to buy options. By hedging, however, you are increasing the cost of the trade
3) I\’d suggest you take the NSE\’s value
Sir,
In 24th August 2015 practical example, It has been clearly mentioned that Deep Otm options have 0 delta and hence, Thought underlying decreased, The premium increased because of Volatality increased.
In \”Trading Options greeks\” book of Dan Passarelli – It has been mentioned that with increase in Volatality – Delta of Otm options increase, Delta of Itm options decrease.
Sir the above two are contradictory. Can you please clarify Sir ?
Deep OTM have \’near 0\’, delta. It usually hits a low, non-zero number and stays there until the stock moves. With the increase in volatility, the premium of both call and put increases, implying the increase in the delta. I think what the author means is that the increase in OTM delta is higher than the increase in ITM delta.
hi Sir,
You have explained that \”The Vega of an option measures the rate of change of option’s value (premium) with every percentage change in volatility \” which means change in premium w.r.t change in volatility in underlying is captured by Vega.
But towards the end of this chapter you have also mentioned that \’With increase in volatility, the Vega of an option increases (irrespective of calls and puts), and with increase in Vega, the option premium tends to increase\’.
My doubt is regarding \”With increase in volatility, the Vega of an option increases \”- Does Vega itself changes with change in volatility or Vega just capture the premium change w.r.t volatility change in underlying?
Yes, vega too changes with change in the underlying prices.
Av=annualised volatility is HV and AV are same.If not. As you said HV is the realised volatility of today where will we get HV for today.
The historical volatility, when calculated on a daily basis and expressed in a yearly format becomes annualized volatility.
India vix is currently at 26 is it for current may expiry or yearly expectation what does this number signifies.
This is the expected volatility for the month of May, expressed on an annualised basis.
Sir till Vega learning was going smoothly here everything become hard to understand.
1.HV and AV are the same or
2.HV and India vix are same.If not were to get historical volatility.
3If I have to buy 12000ce should we compare IV of this strike with HV or India vix.
4.How important is it to consider IV of individual strikes.
1) HV = Historical Volatility, AV = ?
2) No, HV is the realised volatility of today (which becomes historical t\’row). India ViX is the market\’s expectation of realized volatility
3) India ViX is fine
4) It does make a difference, so I\’d suggest you do.
Hi,
Where Can I get Historical IV of nifty options for each strike price.
NSE option history does not have this data.
Thanks,
Vinod.
That can be a bit tricky, you should probably try checking with – https://sensibull.com/
Hi,
I transferred 23k to my zerodha account and bought shares worth Rs 22,988. Now my withdrawal balance is showing negative 24.85. Why is it so? Please explain.
This is because of the applicable charges.
Thanks for clarifying, so from the current profit/loss which is shown in my holdings, no further money will be deducted from that for this particular transaction upon selling the shares.
Yes, all charges are mentioned here – https://zerodha.com/charges
Thanks
Typo… It should be \”movement\” and not \”moment\” in the line-
\”This means, irrespective of the move in the underlying the moment in the option premium will be very restrictive.\”
Thanks for pointing that, Dipen 🙂
Hi Karthik,
I have seen a study \”Historical Volatility\” in Kite. Is this study different from what we are discussing here?
The values shown using this study differs from that in nseindia.com .
If they are different, can you explain the difference. Thanks in advance.
Thanks
Srikrishna
I need to check that particular study, Srikrishna.
And also please do check
1) \”Standard Deviation\” study available in Kite. Is this same as what we discussed earlier?
2) Above this doubt, I have asked one about \”India VIX\”, plz clarify that too.
Thanks
Srikrishna
1) Std Deviation is the same
2) Guess I had clarified about India ViX. Can you repost in case I missed answering it? Thanks.
Hi Karthik,
When I google about \”India VIX\” , I see that this statement below:
\”A negative correlation between India VIX and Nifty, VIX tends to drop when Nifty goes up, and vice versa.\”
I didn\’t clearly understand this stmt. How is \”Nifty going up\” and Volatility drop has a relation? Similarly when \”Nifty going down\”, how is this related to \”Volatility increasing\”.
Thanks
Srikrishna
They tend to compare the Nifty Index with India ViX. ViX is also called a fear index, goes up when fear in the market shoots up or in other words, the markets go down. Hence the -ve correlation.
But since \”India VIX\” is related to volatility and volatility exists in both the directions of the market. So, when Nifty goes up, why is volatility index \”India VIX\” decreasing?
I hope you got my point.
Thanks
Srikrishna
Hi Karthik,
If an index (or) a stock price value is increasing and also if volatility is increasing, then it does make sense that the premiums can be increased, since there is a high probability that the Call Option OTM options tend to become ITM soon rather than later.
However, when the same price value is decreasing and the volatility is increasing, in such a case, the Call Option ITM option might become ATM (or) OTM options soon. So, in this case , if you take any particular strike, my thinking is the premiums should decrease.
But, instead of decreasing, whenever the Volatility is increasing (so as Vega), the option premiums are increasing irrespective of the direction. So, why is the Black & Scholes algorithm designed in this way? Any particular point I am missing here?
Thanks
Srikrishna
Agreed to some extent, but then the direction of the market is an output of human judgment. Volatility and its impact on option premium is the same across both CE and PE. Think about it, an increase in vol means more change for the options to transition from OTM to ATM.
Agree with this \”the direction of the market is an output of human judgment\” and also with this to some extent \”an increase in vol means more change for the options to transition from OTM to ATM\” but there is an equal chance that \”an increase in vol means more chance for the options to transition from ITM to OTM\” even.
However, as you mentioned earlier, the direction is a human judgement, and since it\’s not possible to act on the same in different ways, they would have generalized the premium increase or decrease when volatility increases or decreases respectively.
But, I got , what you were trying to explain. Thankyou:)
Thanks
Srikrishna
Welcome, Srikrishna! Good luck with Options 🙂
How to find implied volatility is increased or decreased?
Refer it with the historical volatility.
while comparing IV and historical volatility, which IV should I use as different strike prices have different IVs.
Look for the ATM strike, Jasdeep.
I have recently started trading in options. However still not clear about option writing. I can’t see any way on zerodha kite platform how I can create / write an option . In practice Is it same as selling an option be it call or put.
Its quite straight forward, Sanjeev. Click on the option you want to sell and click \’Sell\’ and hit enter, with this, you would have sold/written an option.
Hi Karthik , Thanks for a quick reply , so what you are telling me is that writing an option is basically same as selling an option . However my query is bit more detailed . When we select any option in the watchlist we get already listed options at various strike rates with CE / PE option. Who creates these options at different strike rates ? let\’s say Infy options are available upto strikes of 960 ( as on 2nd feb , 11:20 am ) but I want to create an CE/PE option at strike of 980 , so can I do that as a individual trader . If not who creates these options in the system ?
Yes, Sanjeev both writing and selling refer to the same thing. The strikes are dynamically created by the exchanges and vary based on the movement in the stock price.
Just awesome!!!!
Keep going!
Sir, what is the effect of Vega on premiums on the expiry day(intraday)…is it completely negligible?
The effect of Vega reduces as we approach expiry. However, it certainly is not negligible 🙂
Dear karthik Sir
There are 2 implied volatility (call side and put side for same strike price).
How should do 1 implied volatility (for analysis)?
I think we should find out the average of implied volatility.
Please reply me.
I know it is difficult to me write english please ignore if I don\’t write english properly because I\’m familiar with hindi and also don\’t understand english properly but your expression is so good so I read your articles 👍
Nayak, yes, ideally for a single strike, the IV should be common across both CE and PE. However, this varies because of the demand and supply situation.
Thank you sir,
Can you talk in hindi ?
It will useful for my other query.
I can understand but speaking in Hindi is quite bad 🙂
To mai ab se agli bar aapko apne query hindi me puchhunga.
Aap jitna ho ske pls hindi me ans. Krna☺️
Uske baad n ho paye to english me dena.
It will helpful for me to understand all your priceless knowledge and thank u for answering me.
Acha, mai koshish kar than hoon 🙂
Dear Karthik,
1) Where can I get a consolidated graph of Historic Volatility,Implied Volatility and Price data for a stock or Index?Or is there a way to get this consolidated data?
2) We say that when Implied Volatility increases then the option prices also increase. I would like to understand whether it is Call option or Put option whose price will increase. This will help to take decision whether call needs to be shorted or Put?
Regards
Chander
1) Maybe you should check with Sensibull if they have this or intend to put this up anytime soon
2) With the increase in implied volatility, the premium of both call and put option increases.
Hi kartik sir,
I recall you telling that when volatility increases nifty falls as both have inverse relationship. Today I have observed that while vix was rising even nifty was rising. Now how do we interpret this?
Rgds,
Shyam
Its not always the case, Shyam. Most often when volatility increases the fear is high and therefore Nifty falls. However, there could be instances when Nifty marginally increases and vol also increases.
Dear Karthik
Is volatility measured per day ?Not sure how to put my question but let me try to ask you with an example.
Let\’s say I have the India VIX chart open ( live ) for 5 mins chart and I want to short option based on the fact that volatility is high ( it can be Nifty or any other securities ). Do you think it makes any sense to trade volatility on a smaller time frame or is it only good on higher time frame ?
Thank you
Oh yes, it certainly does, Ron. All option greeks are on a continuous basis and not really on EOD basis.
Thank you Sir !!
Welcome, Ron.
Hi Kartik
What about Theta? Is it daily basis or continuous?
All greeks, including theta work on a continuous basis.
Dear Karthik,
Sorry to bother you again with my not so sensible questions. But I would appreciate if you can please let me know the following.
1. Do we have to calculate HV and IV on our own before making a trade ? Or is it readily available in the Zerodha platforms ?
2. Does Zerodha offer charts of HV and IV ( of all indexes and individual stock\’s options ) in their softwares ? or do we have to work with the number\’s provided for HV and IV.
3. How important is it for us to look at the HV and IV chart before making a decision to buy or sell ? or is it possible to make our option strategies decision based on the numbers available on HV & IV ?
Thank you once again Sir !
1) You need to calculate. However, you can check https://sensibull.com/ as well
2) Nope
3) I\’d suggest you look at volatility, Ron.
Thank you very much Karthik.
Happy learning!
Dear Karthik first of all we\’re grateful to you of teaching one of toughest things in market- Options in a layman terms & given us wisdom more than what we deserved. Although I\’m physics Ph.d students so mathematics isn\’t big deal but still your explanation had given more deep insight. I\’m also a client of zerodha from last one year. My few questions are as follows
1) From last few chapters especially theta & volatility, does it mean that we should not be a buyers of options at6 all? Or if we buy then kindly when & at what favorable conditions.
2) Since options sellers required huge margin, it\’s often touted in market as big players cup of tea while mostly retailers are buyers? Is it true to this rumours ?
3)Zerodha by far till now has been very great experience in investing & trading but there\’s sometimes rarely little problem arises in options trading as technical glitches don\’t show the premium movement. How to deal if such situations?
Thanks a lot
…Your kindness
1) Not really, Harsh. Buying options really depend on the situation. A lot of time to expiry, momentum in the stock expected, then why not? However, option sellers, especially deep OTM have a better chance to retain the premium
2) The margins required is similar to that of futures, and as you may know many retain participants trade futures. So this is baseless 🙂
3) This is probably because there is no liquidity, not a technical glitch 🙂
Thanks a lot Karthikji for your kind reply.
Only few more query plz regarding qestion no.2 above…
1) Actually I read somewhere probably an article in rediff that 80% of traders In India trade in options & remaining in Futures & Intraday, although in that amount also buyers are more than sellers which points to retailers participation.
2) Even Sebi also raised red flags last year that the ratio of India\’s Derivatives to Cash segments is one of highest in the world. The number reads 14 next only to Korea. Which again points to retailers participation in buying options or futures segments. In that report if my memory serves well then it\’s been mentioned that Options sellers are mostly HNI\’s ?
It\’s due to above mentioned reason options buying seems more depressing than sellers because as you taught us has natural statistical edge…
How much truth to above query.
Kindly sorry for long query again…
Your kindness
1) Yes, I\’d kind of agree. There are more people trading options than futures
2) I remember that number, but I think its flawed because the derivative volumes are notional. But yeah, option selling requires higher margin, probably that makes one believe that option writing is for HNI.
but i just dont get it …does it make sense to buy such far out of the money(1000 to 1200 points away) strikes and if not why people buys it? thnx….
Nope, buying far OTM is not a great idea, unless you have some concrete reasons to do so.
Please check whether my understanding is correct with volatility –
1.If we calculate daily volatility(SD) of any stock based on historic 1 year data let say we got 3%, so meaning is, it can either go up by 3% or can go down by 3% on next day. We have to predict the stock direction based on our analysis, it can be technical analysis lke patterns,Prior trend,S&R.
Now Question –
2.If we see 24th Aug. example\’s option chain screenshot, all ITM Put options premium increased and What is happen to put OTM (since screenshot is not visible) with respect to volatility and Delta. Can you please explain in detail.
Thank You.
1) Yes, thats right
2) This is because of less time to expiry. As you move closer to expiry, ITM/ATM options turn more sensitive to than OTM options.
It is being said that from 1st Oct. derivative market timings will be increased till 11:55pm.
But derivatives are based on underlying that is equity market, so how derivatives will be traded if spot market is close.
There is no official circular on this yet, Arun. For this reason, I\’m assuming the price won\’t deviate much from the spot. However, in the case of an overnight news on the stock, the derivative market will take lead and the stock would catch up at the opening.
Dear Karthik,
Dude I am really big fan of u..!! Master blaster….Man 😉 Pro 🙂
Having query regarding volatility :
As per today\’s 28-Aug-18 data from NSE site Maruti suzuki\’s Previous Day(27th Aug) Futures Volatility is 0.0119, Current Day Futures Daily Volatility 0.0125 and Futures Annualised Volatility is 0.238. i.e. current volatility is almost half of annualised volatility.
as i have read in some module that higher the volatility, higher the premium and premium will cools off followed by volatility cools offff.
can we consider that lower the volatility is lower/cheap price of any counter [maruti in this case] ?
can we look up move in maruti as per annualised volatility?
Thanks for the kind words, Mayank 🙂
Yes, you can consider trading volatility either ways. But, if you are looking at buying options due to low vol, then remember you are also moving against the time decay factor, which you may have to avoid.
Thanks brother…!!!
now take maruti as example…
For sep month expiry having much time and time decay shudnt effect that much i think and can buy call option,?
Another view if we consider time decay …so shud we make bull put spread/ bull call spread or can we sell naked put to eat time decay?
Yes, when there is ample time to expiry, you can think of buying an option. You can take any position which suits your directional point of view. Generally speaking, if you are right on the direction, the naked option gives you maximum bang for your buck 🙂
Hi Sir,
I have gone through the volatility chapters my question is using the STDEV formula can we calculate the range for one single day for nifty, any indices or a underlying for eg any stock if yes pls explain.
Yes, you can calculate the range for the day for any index or stock.
Hi Karthik,
Something strange happened today(31/05/2018), I was wondering if Volatility was a factor that the Premium went the other way around.
So i was holding HDFCBANK CE 2140 Strike and at 3:00 pm
HDFCBANK in Cash Market was trading at 2127.00 and the HDFCBANK18JUN2140CE at 46.00 ,
in a matter of few minutes by 3:18 HDFCBANK shot up to 2160.00 , crossing my strike price ,so i anticipated by option premium to go beyond 60 ,with Gamma turning 0.5 , but there wasn\’t much movement in the premium ,it just fluctuated around 46.
Would be happy to understand why this happened?
I don\’t see an option here to upload a screenshot to help you understand my point.
Laukik, this was literally 15 mins to expiry. YOur option was ITM by 20 Rupees, but according to you it was 46, so even at 15 mins to expiry, you had an awesome premium on your premium 🙂
Hi Karthik,
But this was June Series Call option i was holding which still has another 4 weeks left to Expire.
Ah, I see. Sorry, I thought this was May expiry. In this case, the premium would have been limited by liquidity and vega.
\” This can be a bit overwhelming for newbie traders. It can be so overwhelming that they quickly rebrand the markets as ‘Casino’. So the next time you hear someone say such a thing about the markets, make sure you point them to Varsity ☺. \”
Certainly… I have already directed few friends of mine to read Varsity, I have made 3 guys buy your Rupee tales. Awesome work .. seriously 🙂
Hahah, thanks for that Kiran!
Markets is chaotic from outside, buy quite systematic from inside 🙂
Hi Karthik… in the prevailing market where the volatility isn’t that high if, we sell call option at higher than 1SD the premium collected will be very low. Do you think it is a better idea to do a short startngle at far OTM option on last Friday before expiry? Say, for example selling call at 10800 and put of 10100 with Nifty spot being 10500.. this way I think we can pocket larger premium and also have some cushion from premium of call option if, market falls drastically and volatility increases as days left to expiry will be just 4 and theta will also act as a saviour to an extent.
Yup, this works. Its just that the margins blocked will be way higher, but this also comes with a \’certain\’ amount of comfort. So margins blocked to returns generated may not make economic sense. It\’s really up to you.
Also, you may want to consider strikes lower than 10100 for the puts.
Thanks a lot Karthik. Margin obviously is way higher but, for novice like me comfort is bigger ticker for now. Also, when you say strike lower than 10100 for puts you mean something like 10000 / 9900 correct (for present spot of 10,550 or so)?
Again all your help and knowledge sharing is highly appreciated.
I understand that, Vivek. You need to be comfortable with the position you hold, only then will you gain conviction to hold. Else the chance of messing up is quite high. Yes, I was referring to strikes lower than 10000 like 99900. Keep learning 🙂
Hello Sir,
1)In one of the above queries, you have responded like this – \”Absolutely, Vega for ATM is the highest. It is quite natural, as ATM options sit on the fence. It can swing either ways…so its kind of fickle and volatile. However, OTM and ITM have already taken sides and therefore the volatility is lower for these options.\”
But as per volatility smile, the IV is the lowest for ATM. Can you please clarify.
2)To find out whether the volatility of nifty is high or low, can we compare the historical annual volatility with the VIX ??
3)To find out whether the volatility of any stock is high or low, can we compare the historical annual volatility with the IV of the particular strike ??
Thanks in advance Sir.
Best Regards,
Aishwarya
1) You are comparing the IV with Vega. Remember, Vega captures the change of premium with the change in vol
2) Yes, you can. For a quick estimate, you can also compare the current day vol to historical vol
3) Yes, as long as you are comparing this with the ATM strike, OTMs and ITMs can give you biased opinion 🙂
Hello sir,
So far we have read, premium = intrinsic value + time value.
The intrinsic value is how much money we would make provided we exercised the contract today. But practically, we cannot do this on everyday and we need to wait till expiry. So the above formula works only on the day of expiry.
So, on all the other days,
premium = (delta x change in underlying) + Time value + (vega x change in implied volatity)
Of course, gamma is included in the above in the form of change in delta.
I really cant express how grateful I am to you. All that I can do is to include you in my daily prayers. God bless you and your family.
Thank you so much sir.
Aishwarya
Wow! Thanks for the very kind words, Aishwarya 🙂
Yes, that equation sort of makes sense, but please do backtest for accuracy. By the way, time value should be deducted.
according to what you have stated here, % change in premium of NIFTY18MAR10200PE should be less than that for NIFTY18APR10200PE for similar change in IV.
However on comparing changes in premium of these 2 options in current month I found quite THE contrary!!!!!!!
premium of NIFTY18MAR10200PE FROM 27/02/18 TO 07/03/18 rose by 221.2% while premium of NIFTY18APR10200PE for similar period rose only by 132.4%
Please explain where am I getting it wrong???
The change in premium is applicable only from Vega perspective. However, you will have to look at all the geeks in perspective – the time to expiry (theta), delta (speed of mkts), and everything else which acts on the options. You cannot isolate just one geek.
Hello sir,
In the above chapter, you have stated \”The effect of Vega is particularly high for ‘Out of the money’ options\”.
Is it because, the delta of ITM and ATM are higher, which affects the premium equally, in turn reduces the effect of vega on these options
or
the vega as such has higher impact on OTM options.(Like delta has higher impact on ATM and ITM). Kindly clarify.
Thank u so much sir.
Aishwarya
This is because with increase in Vega, there is a greater chance for the OTM to transition to ITM, hence they react quite rapidly.
Hi Karthik,
Really nice piece of article on the events of August 24, 2015.
Is there some instrument or way to trade Volatility in the markets on a day to day basis.
I tried searching for India VIX Future contracts but they have very poor liquidity.
Any suggestion will be helpful.
Regards
Kulbir
The best way to trade Volatility is via options. India ViX is not tradable anymore.
Hi Karthik,
Thanks for your quick reply.
As you may know that when markets open during the morning, there is a spike in volatility for the first 30 to 45 minutes after which the volatility dies down.
I was exploring the idea of buying options of Nifty with delta close to zero in the morning and then selling them before the morning volatility dies down. Before proceeding with this idea I wanted to collect some data and also take some suggestions from an experienced trader like you.Please let me know your opinion on this.
Regards
Kulbir
It looks like a good idea, Kulbir. But my concern is that for deep OTM options to react ( since you want to consider delta os 0), the increase in volatility has to be really high, which may not be the case at the opening.
However, this should not hold you back from collecting data and moving further also if you don\’t mind, do share your results with the community here. Good luck!
Hi Karthik,
I understand the concern about the very deep OTM options. Just to get the probability in my favor I have thought about buying either deep OTM Calls or Puts(depending on if the market opens up or down). Will try not to go very deep OTM as liquidity might also be a concern.
Yes I will definitely share the data if I find it useful.
Regards
Kulbir
Good luck, Kulbir!
VIX is calculated based on OTM options order. That is the reason OTM options price changes more than say \”X\”% daily ? Want to know VIX impact only, other factors like theta, delta also matter but want to know vega effect only.
Vega effect on?
Vega effect on OTM strikes.
Increase in vega increases the option premium.
A decrease in vega decreases the option premium.
HI Karthik,
\”Thank you\” is very small word for the kind of knowledge and service you are doing for the financial world. You are teaching how to fish rather than giving fish. Hats-off for your efforts and dedication towards educating us and guiding us in proper direction.
Coming to this article, I have few doubts regarding Vega. Could you please elaborate the impact of Vega on deep OTM option?
Referring the August 24, 2015 scenario
1. I really don\’t understand why the option buyer wanted to pay more premium when markets were in deep correction and also the other options below 8650 CE were available with lesser premium?
2. If high volatility increases(High Vega) the premium, why did the options below 8650 CE started losing the value.
Thank you in advance.
Thanks for the kind words, Subbarayudu 🙂
1) It is not really about what the buyer wanted to do – its more about why the premium of a call option shot up on a day like that. This is a counter-intuitive and can only be attributed to the increase in Vega
2) This is because they had a higher delta, which is more reactive to the changes in the spot.
1. Pulp fiction is over rated
2. This chapter was a bouncer… should have written it within 3-4 chapters like delta and gamma. My brain is unable to understand this!
1) Pulp Fiction is very similar to markets – people are bullish and bearish on the same stock at the same time 🙂
2) I have, starting from chapter 15!
Hi Karthik,
To Predict volatality of a stock
1.Where can i get GARCH Tool,pls share link
2. What are the input parameters required to use GARCH(1,1)
3.Where to check ANNUALISED IV of a stock,pls share the link.
Thank You ಕಾರ್ತಿಕ್ ಭಾಯ್!
You need to build one yourself – you can do this on excle if you know the math behind. Unfortunately, I\’m not aware of any tool available online. I\’m not an expert on GARCH, so my inputs is wont really matter 🙂
is it proper to apply normal technical analysis rules to the INDIAVIX candlestick chart and trying to predict whether volatility is going to increase or fall?
No, it is not a good idea to apply TA to INDIAVIX. I\’d suggest you look up on few statistical techniques like GARCH(1,1) for predicting volatility.
Can we conclude that the volatility of stock has increased from nse option chain IV. Suppose IV on 13 dec. Is 33% and on 15dec for same strike it is showing 50%.Will this implies that volatility of stock has increased from 33 to 50.
Yes, this is exactly what it means.
Thank You very much.
One more doubt suppose currently implide volatility of sbi for 310 strike is 30% and it\’s annualised volatility is near about 44% does it means that volatility of sbi is low than it\’s historical volatility and one should look at buying the premium (after considering theta)
Yes, this is true purely from a volatility perspective. However, you may want to consider other forces at play before you buy the option.
Thank you very much
There are 2 stocks A and B.
The IV of A for its ATM strike for particular expiry is 23.
Similarly the IV of B for its ATM strike for the same expiry is 35. Can we say that B is more volatile than A?
Thank you
Yes, you can.
Thanks sir
Hello sir
1.How could we know if implied volatiliy of a stock is low or high?
2. How could we know if the implied volatility of a stock is going to increase or decrease?
3. Does a naked option buying is mere a speculation and not a trading?
thanks
1) By comparing it with the historical volatility
2) That is your trading call
3) Again depends on your trading logic.
HI Karthik ,
On 02-10-2017 I HAVE CHECKED THE NSE SITE FOR STRIKE @ 9700 (CE) THE IV IS 6.39,and the under lying is trading @ 9786(Nifty Spot Price) . My question is what would be the IV for the same strike price if nifty trade @ 9746 and 9796 ?. If you have a solution please let me know the calculation process .
Thank you for the efforts !
It is hard to estimate the Vega versus spot price. Vega increases when panic on the street increases.
Is there any mechanism available to estimate the Vega ? .
and (IV = Vega) ?
You can use this for both – https://zerodha.com/tools/black-scholes/
what if option underlying is falling continuously assume by 30% and volatility increased by 40%
1. how will CE and PE option premium react?
This also depends on the time to expiry. If there is ample time to expiry, then the fall in price has a greater impact on premiums compared to volatility. On the other hand, if the time to expiry is less…then volatility has a tad bit higher impact on premiums.
How can we use particular strike price IV for analysis?
1. Will we have to compare with it annual Historical volatility.
2. Or we have to compare it with India vix (but India vix is only for nifty ).
You can compare it with historical IV and assess if the volatility is high or low, based on which you can decide to go long or short on the premium.
where will i get historical iv data?
Unfortunately, its not available on the internet I guess.
when volatility increases, the vega also increases and the premium will also increase.
OR
when volatility increases, vega will also increase and the premium can fluctuate in any direction (high or low).
sir, where am i wrong,
Vege increases when volatility increases, and therefore the option premiums.
sir, i am confused in nifty example;
The INDIA VIX (volatility) was 64% up & as you said there will be increase in premium with increase in volatility,
so why there was decrease in premium of ITM, ATM, OTM options and it went down?
Sir, where am i wrong. please correct me
It really depends on the strike you are dealing with. If the option is far OTM, then volatility does not have much impact on premium.
Hi sir,
On july 14,infosys stock price was down by 5.00(0.51%) even though stock went down all put options(every strike price) lost premium why? and also some of ITM call options gained value….how?
With the drop in volatility, the premiums can lose value.
Thank you again for quick response to the queries and explaining complex terms with superb examples. It is very helpful with the kind of examples you give. I do have few more to ask kindly review it.
1.) Banknifty has a daily movement 200-300 points (usually or in most days), so does more volatility signify that it is more prone to black swan event?
2.) The futures of banknifty are trading at a discount of 33 rs. does it have a slight bearish significance though the technical indicate a strong buy or why is it trading lower than the spot?
Since you have provided with so much valuable information and also good recommendations (fooled by randomness, I like I like).
1)I wanted to add few: Vega isn\’t greek, kappa is( vega is more common though, stumbled upon this on mit openware)
2) What we cannot know by marcus du satoy is also a very good read giving an insight on randomness and other factors.
1) Kind of, higher volatility favors black swan events
2) Not really. Discount/premium cannot be used to estimate the sentiment in the market
3) Vega is a 2nd order derivative – so as far as I know, it falls under the Óption Geeks umbrella. Kappa is 3rd order I guess…and it is also an option geek
Dear Karthik Sir,
Thank you and your team for amazing explanations.
One query outside of this topic. With COIN being introduced, can you and your team please share knowledge on how to study Mutual Fund.
Thanks Tanmay, that is high on priority. We will take it up next.
Should we compare IV of strike price directly with annualized HV or Daily volatility * SQRT(no of days left to expiry) ?
How to guage volatility is cooling off?
You need to compare across similar time frames. For example daily with daily, and annual with annual. When volatility decreases, it suggests a cool off in Vol.
Respected Sir,
My question is general, but I find it relevant to post it in this chapter. I searched but found confusing unstructured answers, please help.
1) Just like India VIX, is there any single volatility index for overall Global Markets? If yes, please provide reference.
2) In addition, please list the few important Volatility Indexes of the major Markets (US, UK, European, Asian & others) in the world for overall volatility heat-map.
[I find your guidance highly helpful, for traders like me, to understand/track the extant/effects of (global) events better, like recent French Elections.]
Regards
James
1) You will find ViX for all major markets, but there is no Vix index that aggregates this as a whole. Or maybe its just that I\’m not aware of this. D
2) Do check this – http://www.cboe.com/products/vix-index-volatility/volatility-indexes
Good luck.
As per superb explanation of change in Vega across time, it is clear that as time passes vega decreases. But what about change in vega across option chain of same expiry. I am not able to clearly deduce that from the text.
However, as per my understanding, Vega of ATM must be higher as compared to ITM or OTM, somewhat forming Bell Curve like Gamma. Am i right?? If not, please correct me.
Thanks in advance.
Absolutely, Vega for ATM is the highest. It is quite natural, as ATM options sit on the fence. It can swing either ways…so its kind of fickle and volatile. However, OTM and ITM have already taken sides and therefore the volatility is lower for these options.
Respected Sir,
Getting few cracks in core concepts, please clarify –
1) The IV of option near 9300CE Nifty (Spot-9119.40) is 10.16%. You told it is IV of strike price (not spot price) – not got this point? How can strike price be volatile, it is fixed at regular price intervals to form an option chain and does not move, it is spot price of underlying that moves, right? So, is it IV of premium of that strike price?? Please help.
Regards
James
When I say strike, I\’m talking about that strike\’s premium. Every strike has its own IV.
Ohh! Thanks a lot Sir!
That was very confusing for me when I used to look at nifty option chain taking the concept of Vega into account. Also, it has become understandable why IV is minimum for ATM strikes & rises as I traversed towards OTM strikes.
No one clear trading concept doubts as efficiently as you do.
Thanks again, Sir.
Welcome, James. Good luck and happy learning.
Respected Sir,
Gratitude for your last guidance regarding the portfolio optimization & beta values.
And thanks for simplifying such advance trading topics for us for laying strong foundation of Model Thinking. Have a few general doubts –
1) When we talk about ‘volatility’ (informally) in day-to-day trading practice, in general, which volatility are we exactly talking about (IV, Historical, Forecasted, …) ?
2) Which volatility has more significance/priority for traders in practical world?
3) What is considered a normal range of India VIX? For example – Nifty50 ranges between 16x to 20x P/E with average of 18x (as per Module 3 Chapter 11). However, now I think its range has practically shifted up.
4) Volatility is high = Vega is high. Does that mean India VIX is high = Vega is high?
5) Is there any defined method/model/process which converts market triggers/news into numerical values for utilization in any sort of formula/model to quantify their impact on price movements? If yes, please provide any relevant link.
6) Does Zerodha offer any programming platform/facility where traders can code their trading models to automate buy/sell using terminal tools & data and market triggers/news, also test and modify it, if necessary?
Regards
James
1) We are generally talk about the current state of volatility which is the Implied Volatility
2) IV
3) Yes, it has. India Vix around sub 15 seems to be the norm these days
4) Yes. But remember, ViX is for Nifty….may not be applicable to stocks.
5) Yes, check for the concept of \’Principle Component Analysis\’. It is a statistical technique, traders use it in markets for the exactly the same purpose that you have mentioned. I have very little knowledge about it
6) You may want to check out Kite Connect APIs – kite.trade
Thanks a lot for your guidance.
Welcome.
Karthik,
I know and remember the day 24 AUG 2015. A doubt popped up giving this a second read. How did the settlement happen on this unusual day? I mean since the option premium increased did the long gained money and shorts lose? Ignore if it is a foolish question. Just out of curiosity.
Settlement would have happened on the expiry day, remember, there is no M2M in options.
Hello karthik,
Thanks for the reply.
I have gone through the chapter 4 again and this is what i understood :
Irrespective of the spot price i can choose the appropriate strike price and in the above case i wish the spot remains at 475 or below strike i.e 500 only then i make money.
But I have a small cofusion…
Please guide
Suppose
Strike – 500CE
Spot – 475
Position – short
If i do not exercise, and square off my position then, what amount of premium received will i be able to keep ?
Can i square off the very next moment ?
Sorry to bother you karthik again.
Thanks in advance.
If you are shorting a 500CE at lets say Rs.15 premium…and the spot expired at 475, then 500CE will have no intrinsic value and hence you can retain the entire premium. If you are short and the option is expiring OTM, then you can let it go without squaring off. However, on the other hand, if you are long and the option is ITM upon expiry…then you are better off squaring off. This is because of the STT, read this – http://zerodha.com/z-connect/queries/stock-and-fo-queries/stt-options-nse-bse-mcx-sx
Hi karthik,
I have one doubt
Kindly clarify
You have mentioned above that \” imagine you want to write 500 CE option where spot is at 475\”
Here
Spot – 475
Strike – 500
CE – call option & writing CE means i am bearish
So.when i m waiting for 500 CE to drop further how could i sell it when it is already at 475 ?
Thanks in advance
Mehul, 500 is the strike, and 475 is the spot. I\’d suggest you start reading from the beginning of this module to get a clear idea.
Hi Karthik,
Is there any website where we can get CHARTS of historical data of volatility of various stocks/index and also screeners where I can screen options based on volatility, say I want to get list of stocks where implied volatility is greater than its highest volatility etc etc
I\’m not sure of any such websites. However, we are thinking about this and may come up something in the near future.
Hy sir,
Thanks for the reply, one more doubt . Implied volatility means expected volatility in future .If 8300 ce has IV of 11.94.
1. IV of 11.94 means volatility in particular strike or spot?
2. IV of 11.94 means future expected volatility then how we get the current volatility..?
3. IV of 11.94 is high or low?
Thanks
sarath
IV means the expected volatility as of today.
1) It is the IV of that strike
2) As I said, it is future vol as of today
3) Compare this with past historical volatility to get a quick idea. This is not the best method but helps you with a give and dirty view.
Thanks karthik….
Welcome!
sir,
is there any software that give live option greeks ..?
Thanks
Sarath
Not sure Sarath!
Dear Sirji, is my observation right? on 14th dec nifty index closing price was 8182.45, and nifty 8100dec pe was 68.70 and volatility around 18%. so theta was 2.86 delta 0.342, vega 6. and the next day nifty close 28 point down 8153. but 8100dec pe was also don by 7 point around 61.35. and volatility around 15%. so my question is why the pe was also down.due to volatility? volatility was down by 3% so loss of 6*3=18 points and theta loss was 3 point. so total loss of long option 21 point aprox. and due to delta around 0.35 the profit was 28*.035 was 10 point resulting to the net loss of 21-10=11 point which worked to 68.70-11=57.70, and it is closw to 57.35 which LTP on 15 dec 2016
Check this – http://www.thehindubusinessline.com/portfolio/technically/when-options-strike/article7596687.ece
Thanks Sirji
Welcome!
Sir I m trying to open the link but it\’s not opening can you provide the link if it is in Trading Q&A.
Which link are you talking about? If its the HBL one, then it is working fine.
Hi kArthik,
Many congratulation for winning Bootstrap startup award.As always i loved your every chapter and contentiously reading your module and yes this help me a lot while doing trading and it always provide me a confidence of thinking in a technical way rather then following instinct.Thanks for such a hard effort.You are doing something out of the box.I always suggest people to follow varsity.
I have certain inquiry.
1: Can i operate multiple brokerage account as i am trading with sasonline but i aslo want to taste your platform.
2.If NO, then what i have to do in order to register with zerodha.
Thanks for the kind words Madhusudan 🙂
Click on the \”Open Zerodha Account\” on the Varsity homepage (you will find it at right bottom) and enter your details. Someone from the sales team will pick up your lead and help you open your account.
Yes, you can choose to trade with multiple brokers…but frankly, you just need one good broker and that would be Zerodha 🙂
why vega is common for PE&CE at same strike?,despite of having different IV at same strike price.what must be low vega value and high vega value for identifying the trade .
IV for the given strike is same for both CE & PE, else you will have an arbitrage opportunity.
I can\’t understand IV same for CE and PE for given strike. But on the Option chain chart it shows different
can you elaborate ?
All else equal the Implied Volatility for both Call and Put option for the same strike is supposed to be the same. Think about it, implied volatility is the volatility that the market expects on an overall basis. Market does not differentiate and allot different implied vol\’s for call and puts. Hence IV is the same for both CE & PE. However, markets are not efficient as we think – the discrepancies in demand / supply, sentiments factors etc act upon the IVs and therefore they tend to be different for different strikes.
Hello Karthik Sir,First of all Thank you for sharing your trading experience with us.its really very helpful for us.Still i m trying to learn Option so please help me and do needful..
I have some doubt related to volatility…Please do needful…I got the volatility data of CEAT of September series 2016.
CALL 30.31
PUT 35.12
HV 41.29
From these data, there would be any help to take decision before initiate trade if so please share your knowledge..
You are welcome Avinash.
Just with these many data points it is not possible to identify a trade. You will have to dig deeper, for example identify if the historical volatility is higher or lower compared to the current volatility…if its higher, maybe you want to sell an option..or if its lesser, maybe you want to consider buying an option. So this thing needs more digging up.
Hello Sir, I just wanted to clarify is my approach right. If I want to buy a Call Option, I will first check Volatility Cone of a given underlying and if the volatility is near -2SD and if GARCH model predicts the future volatility to increase in next few days before the expiry.
Bingo! That is exactly how one should go about before buying naked options 🙂
Thank you very much sir 🙂 I would be really grateful if you could share GARCH process excel sheet or R programming file, I tried making it myself but it was really difficult to understand. I wanted to know one more thing, Sir as you had said in Future Trading module to predict movements of NIFTY, one should have knowledge about general Indian Economic activities so from where can I gain this knowledge.
Will try and upload the GARCH models, but cannot really commit a timeline for this.
To keep tab on general macro economic events, the best source is your daily news paper!
Thank you very much sir 🙂
Welcome!
I\’ve read all the chapters of this module and made a strong conception upon Option. But got trapped in Vega section. Here I\’m not able to relate vega with volatility. Can Vega relate with volatility and time as in the link – http://www.theoptionsguide.com/vega.aspx
Please correct me where I\’m wrong!
Vega captures the change in premium for the given change in volatility! This is quite straightforward. Is there any particular part which you found confusing?
Yes. On 15/07/2016 Infy had down a 1000 point. A huge volatility. Can you please elaborate each greek change with the volatility depending upon ITM , ATM and OTM option. I have
I\’ve done that already! Please start from this chapter – http://zerodha.com/varsity/chapter/the-option-greeks-delta-part-1/
Sorry, Karthik to bother you. I was thinking from only one side perspective which jumbled me.
And thanks for you quick reply.
Welcome!
Dear Karthik, IS the following comment addressed in your new articles ! Can you direct through a llink . Thanks ….Realized volatility matters especially if you want to compare today’s implied volatility with respect to the historical implied volatility. We will explore this angle in detail when we take up “Option Trading Strategies”.
I\’ve addressed this is in various bits across the modules. However the most concrete way of dealing with this is by setting up volatility arbitrage trades, which is still not explained in Varsity.
In chapter 19.2 you have mentioned that vega will be calculated on change of each % of volatility..so here volatility is implied volatility or india vix???
India ViX is the implied volatility of Nifty. Yes, one needs to look at the IVs to derive the Vega.
If is it so then INDIAVIX data should match with IV in the Option Chain data of NIFTY(For ATM case). Tell me if I am wrong here .
Actually I also want to know that how implied volatility is calculated using the order book of Option data.
Hmm, it should I suppose. Although I\’ve never really checked this. The calculation is quite complex, you can find some insights here – https://www.nseindia.com/content/indices/white_paper_IndiaVIX.pdf
Dear kartik sir
If u don\’t mind Can u please give a clear example of how to calculate vega as i didn\’t understand clearly from the above example given by you…i mean vega should be calculated on daily implied volatility or what?? Still confused… please give one more example in detail…sorry for giving you trouble??
Vega as such is derived from the B&S model, check this post to understand the framework – http://zerodha.com/z-connect/queries/stock-and-fo-queries/option-greeks/how-to-use-the-option-calculator
Hi Karthik ,
Can You please help me in getting the source of the data mentioned in your Volatility Cone excel sheet.
Thanks and Regards
Devenndra
Guess NSE India has all the data you\’d need. Check this – https://www.nseindia.com/products/content/derivatives/equities/historical_fo.htm
Karthik,
How do the Greeks automatically keep changing the price of an option? Is there a market maker? Also, about the news article you mentioned, did the premiums automatically adjust or there were people who bought or sold to affect the price?
There is no concept of market making in India. However there is a fairly good arbitrage environment, mainly driven by HTF algos. I suppose they are responsible for getting these greeks and premium aligned and on track.
Dear Karthik – I read this somewhere \”Usually out of the money options have a higher IV, the trade typically could be if it is much above the MEAN IV, it could be a good time to sell them and much below the MEAN time to buy.\” My question is what is MEAN in the sentence. Request if you can please explain ? Thanks.
Mean = average. So if the historical average volatility is 20% but today the volatility is 30% then you can sell volatility by employing a suitable strategy. Likewise if the volatility is lesser than average, then you can buy it.
Thanks for the detailed explanation. 🙂
Welcome!
Sir, today on 12/02/2016 IOC declared its results for Q3. From the options chain, it is observed that there is a +ve change in the premium of its CE 360 to CE 410 but at the same time there is +ve change in the premium of its PE 340 to PE 420 ……… Can you please explain the reasons for this +ve change in both CE as well as PE when the underlying security shows a decline of 2.06% in spot? …….. Is it because of the liquidity due to very low volume in IOC options or is it because the Q3 results were declared by IOC at the fag end almost after the trading hours of today?
SEE THE CHART IOC OPTION CHAIN
Yup, as I said it\’s because of increase in volatility!
This is because of increase in volatility! Check the chapter on vega for a detailed explanation.
Hi bro,
From your experience, do you think greek (other than delta) really affects for interday trading?
Thank you 🙂
For intraday Vega and Delta matters the most.
Vega?? lets say I buy now and sell within few minutes to max 2 hours. will that have any affect?
Thanks bro
Volatility can vary at a blink of an eye 🙂
oh I see. Thaks bro 🙂
Welcome!
Volatility changes based on the orderflow… Does it mean that increase in OI in NIfty options a reason for increase in IV of respective options?
Not sure – I;ve never really studied the correlation between OI and IV, so cant really comment.
how to use vega in calculation premium. you have explained use of delta and gamma in calculating option premium.
but how to use vega value that we get from B&S calculator?
Well, vega can be used pretty much the same way as delta..I guess you got the math right in your previous comment with Delta & Theta.
Hi,
I found the article very interesting and I thought I should leave a comment for a few reasons.
First of all, I am a big fan of Tarantino. Pulp fiction, Reservoir Dogs, Django Unchained are my favourites. I can recite a number of dialogues from the movie. I love them
secondly, I can\’t forget 24th August as that’s the date the market so spectacularly taught me that I was wrong. I lost over 1.5lac in less than 30 mins of opening 🙂
my 4 months of hard work, crushed by the steam roller in less than 30 mins. In all other times that i had made loss in the past, i had exactly known what I did wrong. Some of the time it was greed, fear in some other or mere sloppiness. But this time I had stuck to the plan, did all the right things, but I had a spectacular fall. It left me confused and questioning my strategy. I haven\’t quite found a method to tweak my strategy to withstand such a fall and therefore haven\’t been trading lot since.
And thirdly, I can relate to the OTM calls refusing to give up value on the day of the crash. I wanted to square off the CALL side to free up capital to repair the PUT side. But the CALL side gaining value instead had greatly annoyed me 🙂
It was Vega in play. Another lesson learnt, humbly.
Market is probably the best teacher! It has unusual ways to teach simple lessons 🙂
I love Tarantino as well 🙂
Good luck for all your future trades – learn & evolve!
Karthik, Would it be a fair comment if i said that IVs tend to work more in your favor when you have bought puts rather than when you have bought calls. I say that based on the following understanding.
1. IVs are represented by the VIX index which is also known as the fear index.
2. Markets fall on fear and Vix generally rises in a fearful market. On the other hand, when there is less fear and more confidence/greed, Vix is generally lower.
3. We buy puts when markets are falling and by corollary from the above 2, Vix works in our favor because it is likely to rise…..
Most of the opposite happens in case of calls. So in a call, we generally dont get help from the IV…..
Would you agree or Have i understood something incorrectly?
Wish you a very happy new year!
I know whatever you said sounds very intuitive…however Volatility has similar effect on option premium irrespective of calls or puts. I would suggest you read this – http://www.thehindubusinessline.com/portfolio/technically/when-options-strike/article7596687.ece
Hi Karthik
Sorry for clobbering you with questions but I really am unable to sort these doubts of mine.
1) I am facing trouble as to when to use India VIX, Annualized volatility given for the Nifty as such and the different Implied Volatility for different strikes in the Option chain. Please clarify
2) Some strikes don\’t have any IV given. What does this imply?
3) As mentioned by you most of the trading in Options is done with Volatility concept as the fulcrum, how to go about it? I googled GARCh as mentioned by you but couldn\’t find anything fruitful.
That would be all as of now 😛
Vivek, keep them coming – its always a pleasure to help!
1) I would suggest you take the annualized volatility and not really the strike specific volatility
2) Not sure why the data goes missing for few strikes
3) Check this for GARCH – http://cims.nyu.edu/~almgren/timeseries/Vol_Forecast1.pdf
Karthik,
I have tried looking on internet for GARCH and Volatility forecasting stuff. I went through the pdf you shared as well but to tell you frankly I didn\’t understand a single sentence. All sounded French to me. Can you please let me know what am I missing or if you could suggest some more basic literature. The best option would be your easy-to-understand lesson on Statistical analysis but gauging limited time availability to you if you could share with me some literature I will be obliged to you. 🙂
The PDFs are best source. The topic itself is quite mathematics/statistics heavy…simplifying it would be a herculean task!
1) can you please explain more about VIX india ?
2) vega is always positive …. volatility be always positive or it can be negetive as well ?
3) vega intereacts with volatility or change in volatility ?
4) vega always appreciates the value or it may depreciate the value as well ?
5) can be consider vega and OPTION-BUYERs friend ,if it always increases the value ?
1) Check this – http://zerodha.com/z-connect/queries/stock-and-fo-queries/trading-india-vix-simplified
2) Volatility is always positive
3) Vega represents the sensitivity of the premium wrt to change in the volatility
4) Increase in volatility (and hence vega) always tends to increase the premium
5) Low vega is buyer\’s friend, high vega is seller\’s friend 🙂
thank you sir ,
continuing question to point 4 .
1) what happens when volatility decreases after we have bought the option ?
2) there are several technical & fundamental analysis methods to predict directional movement …… how the same the be done to predict increase or decrease in volatility ?
3) is it possible to create to vega neutral option pairs ? i assume no, as vega is always positive .but pls do elaborate .
4) kindly elaborate more on \”Low vega is buyer’s friend, high vega is seller’s friend\” .
5) IN THE OPTION CHAIN SHOWN IN THIS CHAPTER ….. CERTAIN STRIKE PRICES HAVE A ( – ) in the IV , means IV not available ….. what exactly does it mean ?
thank you
1) The premiums tend to go lower due to lower volatility. But then the direction also matters…if the underlying is moving quickly then on one hand premiums would go up (due to the speed at which underlying is moving) and on the other, the premium would tend to go lower (due to lower volatility). Finally which ever greek has more significance (in that particular scenario) would dominate the play.
2) You need to be aware of both, check chapter 23, section 23.5.
3) Should be – I will get back to you on this at a later stage
4) Low vega means low premium value…so good for buyers and vice versa
5) I guess so.
Hi Karthik,
Few Queries..
1. What value of vega is considered as high and low for stocks or indices?
2. what values of IV\’s are considered as high and low for Bank Nifty?
For indices – take ViX value. About 17-18% for Nifty is average.
For Stocks and Bank Nifty – you need calculate the daily volatility and convert it to annual volatility to get a quick perspective.
Dear Sir,
(1)Likewise 12-15% Nifty IV can be considered as LOW, I would request you to kindly advise from where Nifty can be considered as HIGH for guidence purpose. ( 2 )Also can I consider above 17% of India VIX as HIGH. Thanking you very much Sir. R.V.N.Sastry
17-18% is the average…anything below is low and above this is high.
How to conclude that the present IV for a particular stock is high or low? Is it based on vega?
For this you need to calculate daily historical volatility and convert it to annual volatility and get a \”quick\” perspective.
dear sir, instead pof calculation ……. can we simply compate the strike rate IV to annual volatility mentioned on NSE website and draw our conclusion ?
Of course you can.
So, if one thinks that there will be an increase in volatility, the prudent option would be to buy an OTM option because-a) the effect of Vega is higher on OTM options and b) since they have a low delta. Also, it would be better to buy an option which has more time left till expiry as the effect of theta will be minimal. Is that right?
Right – when buying options it is important to evaluate the situation based on time and volatility. There has to be more time for expiry, and the volatility should be expected to increases.
In Chapter 5 :
I find the below content…
—————————————————
Important note – The calculation of the intrinsic value, P&L, and Breakeven point are all with respect to the expiry. So far in this module, we have assumed that you as an option buyer or seller would set up the option trade with an intention to hold the same till expiry.
But soon you will realize that that more often than not, you will initiate an options trade only to close it much earlier than expiry. Under such a situation the calculations of breakeven point may not matter much, however the calculation of the P&L and intrinsic value does matter and there is a different formula to do the same.
To put this more clearly let me assume two situations on the Bank Nifty Trade, we know the trade has been initiated on 7th April 2015 and the expiry is on 30th April 2015–
1.What would be the P&L assuming spot is at 17000 on 30th April 2015?
2.What would be the P&L assuming spot is at 17000 on 15th April 2015 (or for that matter any other date apart from the expiry date)
Answer to the first question is fairly simple, we can straight way apply the P&L formula –
= Max (0, 18400 – 17000) – 315
= Max (0, 1400) – 315
= 1400 – 315
= 1085
Going on to the 2nd question, if the spot is at 17000 on any other date apart from the expiry date, the P&L is not going to be 1085, it will be higher. We will discuss why this will be higher at an appropriate stage, but for now just keep this point in the back of your mind.
——————————————————————————————————————–
TO BE PRECISE,
My Question is : If I am PUT or CALL Seller do I need to wait till end of EXPIRY to execute ( Square Off ) My Position?
HOW is the PROFIT LOSS calculated in case of Selling CALL and PUT.
In case of an options seller, the profit is limited to the extent of the premium received. However the loss as you may know can be unlimited. Also you need not have to hold the options till expiry, you can choose to square-off whenever you want.
Thank you for all these useful and concise materials.
Today(18/9/2015) nifty closed up around 83 points @7981, but Sep 24 CE8200 lost 53% closing @8.25
Can u tell me why this happened.
Few reasons –
1) Volatility cracked around 17%…check India Vix
2) Time value (Theta) is starting to act on options premiums
3) OTM option\’s Gamma starts tending towards zero as we approach expiry (although we have another 6-8 days for expiry)..hence the impact of delta (further implies impact of directional movement of underlying) is low.
Hi Karthik,
When can we read on Option Strategies ???
Very soon Sir. Another 2 or more chapters in Options Theory and we are good to start Options Strategies.
What is Series while downloading historical data. I\’m trying to download SBIN data.
The series would be \’EQ\’ for stocks (including SBIN). The reason why you see so many series for SBIN is because SBIN is a Bank and besides stocks, most of SBIN\’s debentures and bonds are listed as well.
Thank you so much! And yes your writings are precious. Very eagerly waiting to read Options Strategies. You won\’t believe I\’ve bookmarked it & check daily if it is there.
Sanket – thanks for the kind words, this is encouraging to all of us!
Another 2 or more chapter and we will be done with Options Theory…onwards to strategies then!
hi karthik . i trade options on the basis of the underlying Stock price movement, by tracking volume and RSI divergences , ADX . and mostly buy naked calls and put options dependent on the stock movement on the b/o for the 2-3 % move. I have studied the greeks on the varsity and this has given me an all new persective on the Price movement, thanks for making it so interesting and easy for anyone to understand, i think you are doing a great job here. Plz request you to also throw some light on LIQUIDITY in stock options and how to check that on any website….cheers
Aks
Glad to hear this Amit – liquidity is an important aspect of trading options, will try and include it in the ongoing series. Thanks.
Hi Karthik,
Today I bought 8000Ce @55 and now eod reach to @75 when I check with zerodha using Black & Scholes option calculator it shows 48.71.
Should I assume this CE is overvalued? or something missing from me.
Mukul – volatility is around 25%, I would suggest you take India ViX values for this.
i tried calculating details for 7900CE
As I said…B&S just gives you the fair value based on the inputs you feed it with.
B&S cal
Hi Karthik, Premium value provide by B&S calculator provided by Zerodha is different from what is posted on option chain in NSE site. Please check and confirm if I m doing something wrong.
Well, the B&S calculator tells you what should be the fair value given the inputs you have fed in. This could be very different from the market value.
Dear karthik
can u pls… tells us in how much time we can expect a full proof module on option topic.
Working towards winding up \’Options Theory\’ by this month end.
Sir, Regarding my above query kindly refer below image
Dear Karthik Sir,
I just virtually take position – long call and long put of slightly OTM strike and difference between two strikes is 100,
Put IV is more than Call IV – Nifty was down by almost 200 points, put premium increased drastically and call premium decreases slowly.
buy sell Qty p n l / no Total profit / loss
Long NIFTY SEP15 7850 PE 175.16 289.95 100.00 114.79 11479.00
Long NIFTY SEP15 7950 CE 181.98 143.25 100.00 -38.73 -3873.00
Net profit 7,606
Call IV is more than put IV – Nifty going up by 100 points, but call premium increasing slowly but put premium decreasing very fast
buy sell Qty p n l / no Total profit / loss
Long NIFTY SEP15 7700 PE 160.20 125.45 100.00 -34.75 -3475.00
Long NIFTY SEP15 7800 CE 180.00 202.80 100.00 22.80 2280.00
Net profit -1195
Kindly let me know what exactly is happening because of IV?
See for IV to have any effect on premiums, the change in IV should be quite large. I suppose the premiums here are moving, largely attributable to delta (directional move).
ok..but when Nifty is going up why not call premium is going up? like u said about OTM strikes, suppose delta is 0.5 and if Nifty moves up by 2 points premium should move by 1 point, and also put premium falling sharply.
I also observed that even if Nifty is moving in upward direction call premium is falling and put premium is increasing, is it because of participants expecting reversal?
Also kindly let me if any virtual trading platform available in India to test options strategies, I think that would be better to test options strategies without actually investing hard earned money.
Thank you
Firstly, OTM deltas cant be 0.5…it has to be a value far lesser than 0.5. Change in premium is dependent on delta, time to expiry, and volatility. If the volatility is high then even if markets are dropping, CE premiums can increase.
No, I\’m not sure of any option trading virtual platforms.
May be volcu.be are the place where we can test our skills…
hi, Karthik, in the chapter above, you have said -\” The effect of Vega is particularly high for ‘Out of the money’ options.\” does that mean Vega is higher for deep OTM options compared to OTM options? as, when i calculate using the option calculator vega is higher for OTM compared to deep OTM.
Madhu – yes, deep OTM options do react to change in volatility – but do bear in mind this has to be a massive change in volatility, like what happened on 24th August. However, such events are not very frequent.
Totally speechless. Wonderful chapter. So one should not go long when volatility is high. Can you please explain the safe volatility value for going long on options.
I assume that change in vega will come in the next chapter.
Can you please give examples w.r.t. the event happening every three months, company results and volatility.
I have noticed last week at the end, option value declined even though there was increase in the stock value, due to volatility came down to normal value. e.g. relcap CE340.
Can you please also give us the list of remaining chapter in this module to know how much we do not know and how much time we shall wait to really restart option trading.
Thanks a lot.
Glad you liked the chapter. Here are the answers –
1) For each instrument the volatility value varies. For example 12-15% volatility for Nifty could be considered low…but Infy 20-25% could be considered low.
2) Have discussed change in vega in this chapter only, the upcoming chapter will contain details on Gamma vs Time, Gamma vs Strike etc.
3) Corporate results and RBI monetary policy are two regular events that drive up volatility
4) Possible – decrease in volatility reduces the price of option premiums
5) The modules is almost coming to end…maybe 2 or 3 chapters more.
Dear Karthik,
Can u please let me know the website wherein we can see the candlestick formation as the market is on. It was somewhere during the Options module or Technical analysis module. Can u please let me know. Regards.
Have you seen chartink.com ?
hi Karthik, so what does a vega of say 12 and the price of a particular underlying moving by INR 10 signify?
Raj – thanks for bringing this point. I seem to have missed this concept all together. I will work on this this right away…
could not get it.
Vega corresponds to which volatility?
Implied Volatility.
hi karthik, awaiting your views on the above query i had raised. was keenly expecting it to be addressed in the recent module. thanks.
My query was: so what does a vega of say 12 and the price of a particular underlying moving by INR 10 signify?
Raj – I did update section 19.2. You will find the answer towards the end of the section..please do check. Thanks.
Awesomeness !!!! Wish I had read this before 16th May 2014 the election results day, I was long and in handsome virtual profit but suddenly the market went down (now I know the volatility dried up) :-).
I wish vega of brining the newer lessons go high and we don\’t have to wait long for next chapter . 🙂
The beauty of markets is that there are always opportunities….it upto you to figure out where it lies!
Will try our best to upload chapter soon 🙂
very true W.R.T markets opportunities knocks everyday 🙂
Thanks again for your hard and wonderful work.
Stay profitable!
Dear Karthik,
Today, for the 1st time, I made a profit of more than Rs.1000/- on Zerodha website since I am client of Zerodha client. Previously, I mistakenly made a loss of Rs. 270/-. The full credit for this goes to u, because of u I was able to understand options very well. God bless and continue this valuable service to us and the society in whole.
I\’m so glad to hear this, hope this is the start of something bigger for you. By the way you need to attribute your success to your hard work and nothing else 🙂
Good luck!
Do you plan to have a module/chapter on mathematical/statistical models in trading?
On the same note, do you use VAR,GARCH,ARIMA etc. and different time series models using a forecasting software like EVIEWS for trading.
Is it a good idea to combine statistical models with technical analysis for trading or are they chalk and cheese?
Rajdeep – a module on Statistical models will be an awesome! Will probably do that sometime in future.
Yes, I\’ve used GARCH models earlier…but these were built from scratch on R and Excel. Have not used any software for this. Also, I\’m not sure if its a good idea to combine TA and stats…never done that before so cant really comment.
Does Vega won\’t have negative value
Nope.